Page 25 - DMGT515_PERSONAL_FINANCIAL_PLANNING

Personal Financial Planning

Notes Solution:
Using the formula above, with P = 1500, r = 4.3/100 = 0.043, n = 4, and t = 6:
×
⎛
A = 1500 1 + 0.043 ⎞ ⎟ ⎠ 46 = 1938.84
⎜
⎝
4
So, the balance after 6 years is approximately ` 1,938.84.
2.3.2 Future Value of Series of Cash Flows

So far we have considered only the future value of a single payment made at time zero. The
transactions in real life are not limited to one. An investor investing money in installments may
wish to know the value of his savings after ‘n’ years.
⎡ (1 r) – 1⎤
+
n
FVofAnnuity = P ⎢ ⎥
⎣ r ⎦
P = Periodic Payment
r = rate per period

n = number of periods
The future value of an annuity formula is used to calculate what the value at a future date would
be for a series of periodic payments.

The future value of an annuity formula assumes that:
1. The rate does not change
2. The first payment is one period away
3. The periodic payment does not change

If the rate or periodic payment does change, then the sum of the future value of each individual
cash flow would need to be calculated to determine the future value of the annuity. If the first
cash flow, or payment, is made immediately, the future value of annuity due formula would be
used.
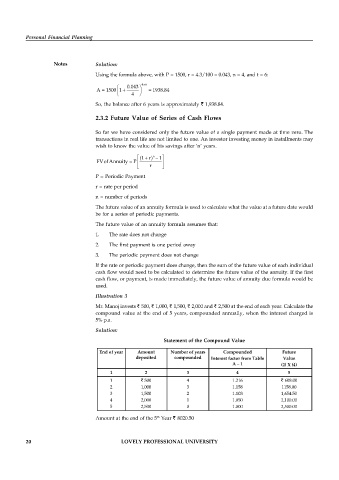
Illustration 3

Mr. Manoj invests ` 500, ` 1,000, ` 1,500, ` 2,000 and ` 2,500 at the end of each year. Calculate the
compound value at the end of 5 years, compounded annually, when the interest charged is
5% p.a.
Solution:
Statement of the Compound Value

End of year Amount Number of years Compounded Future
deposited compounded Interest factor from Table Value
A – 1 (2) X (4)
1 2 3 4 5
1 ` 500 4 1.216 ` 608.00
2 1,000 3 1.158 1158.00
3 1,500 2 1.103 1,654.50
4 2,000 1 1.050 2,100.00
5 2,500 0 1.000 2,500.00
th
Amount at the end of the 5 Year ` 8020.50

20 LOVELY PROFESSIONAL UNIVERSITY