Page 297 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

Notes
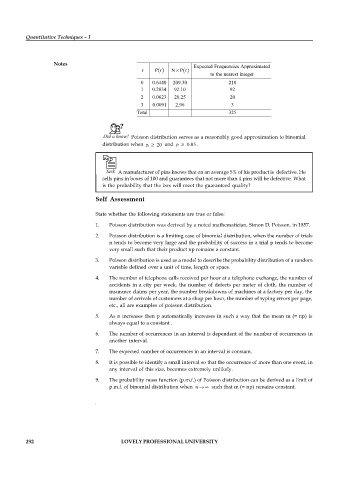
Expected Frequencies Approximated
r P r N P r
to the nearest integer
0 0.6440 209.30 210
1 0.2834 92.10 92
2 0.0623 20.25 20
3 0.0091 2.96 3
Total 325

Did u know? Poisson distribution serves as a reasonably good approximation to binomial
distribution when n 20 and p 0.05 .

Task A manufacturer of pins knows that on an average 5% of his product is defective. He
sells pins in boxes of 100 and guarantees that not more than 4 pins will be defective. What
is the probability that the box will meet the guaranteed quality?

Self Assessment

State whether the following statements are true or false:

1. Poisson distribution was derived by a noted mathematician, Simon D. Poisson, in 1857.
2. Poisson distribution is a limiting case of binomial distribution, when the number of trials
n tends to become very large and the probability of success in a trial p tends to become
very small such that their product np remains a constant.

3. Poisson distribution is used as a model to describe the probability distribution of a random
variable defined over a unit of time, length or space.
4. The number of telephone calls received per hour at a telephone exchange, the number of
accidents in a city per week, the number of defects per meter of cloth, the number of
insurance claims per year, the number breakdowns of machines at a factory per day, the
number of arrivals of customers at a shop per hour, the number of typing errors per page,
etc., all are examples of poisson distribution.

5. As n increases then p automatically increases in such a way that the mean m (= np) is
always equal to a constant .

6. The number of occurrences in an interval is dependent of the number of occurrences in
another interval.

7. The expected number of occurrences in an interval is constant.
8. It is possible to identify a small interval so that the occurrence of more than one event, in
any interval of this size, becomes extremely unlikely.

9. The probability mass function (p.m.f.) of Poisson distribution can be derived as a limit of
p.m.f. of binomial distribution when n such that m (= np) remains constant.

292 LOVELY PROFESSIONAL UNIVERSITY