Page 70 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I
P. 70
Unit 4: Presentation of Data
(b) Construction of a Histogram when Class Intervals are not equal: When different classes of Notes
a frequency distribution are not equal, the frequency density (frequency width) of
each class is computed. The product of frequency density and the width of the class
having shortest interval is taken as the height of the corresponding rectangle.
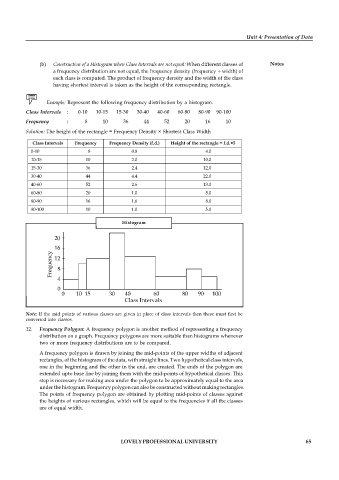
Example: Represent the following frequency distribution by a histogram.
Class Intervals : 0-10 10-15 15-30 30-40 40-60 60-80 80-90 90-100
Frequency : 8 10 36 44 52 20 16 10
Solution: The height of the rectangle = Frequency Density × Shortest Class Width
Class Intervals Frequency Frequency Density (f.d.) Height of the rectangle = f.d.×5
0-10 8 0.8 4.0
10-15 10 2.0 10.0
15-30 36 2.4 12.0
30-40 44 4.4 22.0
40-60 52 2.6 13.0
60-80 20 1.0 5.0
80-90 16 1.6 8.0
90-100 10 1.0 5.0
Histogram
Note: If the mid points of various classes are given in place of class intervals then these must first be
converted into classes.
12. Frequency Polygon: A frequency polygon is another method of representing a frequency
distribution on a graph. Frequency polygons are more suitable than histograms whenever
two or more frequency distributions are to be compared.
A frequency polygon is drawn by joining the mid-points of the upper widths of adjacent
rectangles, of the histogram of the data, with straight lines. Two hypothetical class intervals,
one in the beginning and the other in the end, are created. The ends of the polygon are
extended upto base line by joining them with the mid-points of hypothetical classes. This
step is necessary for making area under the polygon to be approximately equal to the area
under the histogram. Frequency polygon can also be constructed without making rectangles.
The points of frequency polygon are obtained by plotting mid-points of classes against
the heights of various rectangles, which will be equal to the frequencies if all the classes
are of equal width.
LOVELY PROFESSIONAL UNIVERSITY 65