Page 197 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 13: Coefficient of Simple Regression Method

Solution: Notes
Calculation of Regression Equations

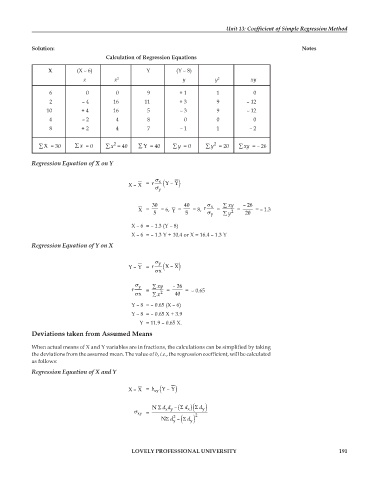
X (X – 6) Y (Y – 8)
x x 2 y y 2 xy
6 0 0 9 + 1 1 0
2 – 4 16 11 + 3 9 – 12
10 + 4 16 5 – 3 9 – 12
4 – 2 4 8 0 0 0
8 + 2 4 7 – 1 1 – 2

2
2
∑ X = 30 ∑ x = 0 ∑ x = 40 ∑ Y = 40 ∑ y = 0 ∑ y = 20 ∑ xy = – 26
Regression Equation of X on Y

σ
X – X = r σ y x ( ) Y – Y

30 40 σ x ∑ xy –26
X = = 6, Y = = 8, r = = = – 1.3
5 5 σ y ∑ y 2 20
X – 6 = – 1.3 (Y – 8)
X – 6 = – 1.3 Y + 10.4 or X = 16.4 – 1.3 Y
Regression Equation of Y on X

σ y
Y – Y = r ( ) X – X
σx

σ ∑ xy – 26
r y = = = – 0.65
σx ∑ x 2 40
Y – 8 = – 0.65 (X – 6)
Y – 8 = – 0.65 X + 3.9
Y = 11.9 – 0.65 X.
Deviations taken from Assumed Means

When actual means of X and Y variables are in fractions, the calculations can be simplified by taking
the deviations from the assumed mean. The value of b, i.e., the regression coefficient, will be calculated
as follows:
Regression Equation of X and Y

X – X = b xy ( Y – Y )

N Σ dd – ( xy )Σ ( d d ) Σ
σ xy = x y
N y 2 – ( Σ d y ) Σ d 2

LOVELY PROFESSIONAL UNIVERSITY 191