Page 260 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Statistical Methods in Economics

Notes
3,600 3,530 3,365 3,400
×
P × P 10 = 3,400 × 3,365 3,530 × 3,600 = 1 = 1.
01
Hence time reversal test is satisfied by the above formula.
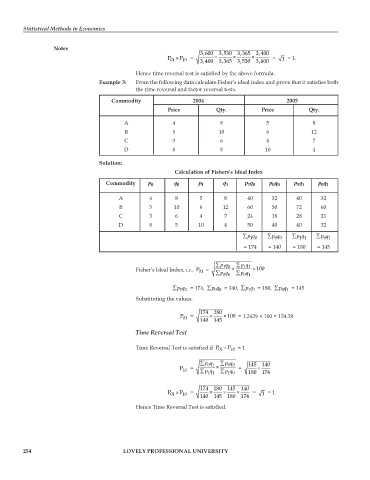
Example 3: From the following data calculate Fisher’s ideal index and prove that it satisfies both
the time reversal and factor reversal tests.
Commodity 2004 2005
Price Qty. Price Qty.

A 4 8 5 8
B 5 10 6 12
C 3 6 4 7
D 8 5 10 4

Solution:
Calculation of Fishers's Ideal Index
Commodity p 0 q 0 p 1 q 1 p q p q p q p q
11
01
10
00
A 4 8 5 8 40 32 40 32
B 5 10 6 12 60 50 72 60
C 3 6 4 7 24 18 28 21
D 8 5 10 4 50 40 40 32
∑ pq ∑ pq ∑ pq ∑ pq
01
10
00
11
= 174 = 140 = 180 = 145
∑ pq ∑ pq
1 1
10
Fisher’s Ideal Index, i.e., P = ∑ pq × ∑ p q × 100
01
00
0 1
∑ pq = 174, ∑ pq = 140, ∑ pq = 180, ∑ pq = 145
01
10
11
00
Substituting the values:
174 180
P 01 = 140 × 145 × 100 = 1.2429 × 100 = 124.29
Time Reversal Test
Time Reversal Test is satisfied if P × P = 1
01
10
∑ p q ∑ p q 145 140
01
0 0
P 10 = ∑ p q × ∑ p q = 180 × 174
1 0
11
174 180 145 140
×
×
P × P 10 = 140 × 145 180 174 = 1 = 1.
01
Hence Time Reversal Test is satisfied.

254 LOVELY PROFESSIONAL UNIVERSITY