Page 261 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 20: Test of Consistency: Unit Test, Time Reversal Test, Factor Reversal Test and Circular Test

Factor Reversal Test Notes

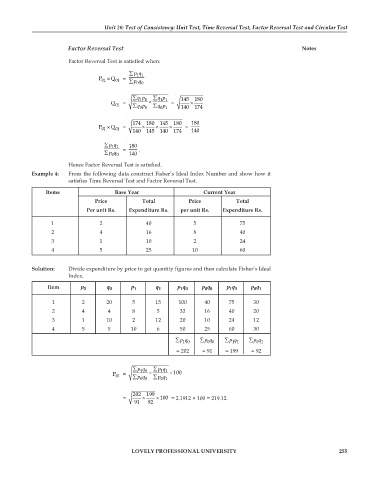
Factor Reversal Test is satisfied when:
∑ p q
11
P × Q 01 = ∑ p q
01
00
∑ ∑qp qp 145 180
11
Q 01 = ∑ 10 × ∑qp qp = 140 × 174
01
00
174 180 145 180 180
×
P × Q 01 = 140 × 145 140 174 = 140
×
01
∑ p q 180
11
∑ p q = 140 .
00
Hence Factor Reversal Test is satisfied.
Example 4: From the following data construct Fisher’s Ideal Index Number and show how it
satisfies Time Reversal Test and Factor Reversal Test.
Items Base Year Current Year
Price Total Price Total
Per unit Rs. Expenditure Rs. per unit Rs. Expenditure Rs.
1 2 40 5 75
2 4 16 8 40
3 1 10 2 24
4 5 25 10 60

Solution: Divide expenditure by price to get quantity figures and then calculate Fisher’s Ideal
Index.
Item p 0 q 0 p 1 q 1 p q p q p q p q
10
00
01
11
1 2 20 5 15 100 40 75 30
2 4 4 8 5 32 16 40 20
3 1 10 2 12 20 10 24 12
4 5 5 10 6 50 25 60 30
∑ pq ∑ pq ∑ pq ∑ pq
10
01
11
00
= 202 = 91 = 199 = 92
∑ pq ∑ pq
1 1
10
P 01 = ∑ pq × ∑ p q × 100
00
0 1
202 199
= × × 100 = 2.1912 × 100 = 219.12.
91 92

LOVELY PROFESSIONAL UNIVERSITY 255