Page 99 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 7: Mean Deviation and Standard Deviation

Notes
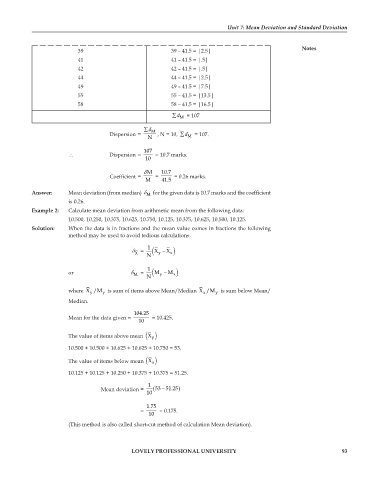
39 39 – 41.5 = |2.5|
41 41 – 41.5 = |.5|
42 42 – 41.5 = |.5|
44 44 – 41.5 = |2.5|
49 49 – 41.5 = |7.5|
55 55 – 41.5 = |13.5|
58 58 – 41.5 = |16.5|
∑d M = 107

∑d
Dispersion = N M , N = 10, ∑d M = 107.

107
∴ Dispersion = = 10.7 marks.
10

δM 10.7
Coefficient = = = 0.26 marks.
M 41.5
Answer: Mean deviation (from median) δ M for the given data is 10.7 marks and the coefficient
is 0.26.
Example 2: Calculate mean deviation from arithmetic mean from the following data:
10.500, 10.250, 10.375, 10.625, 10.750, 10.125, 10.375, 10.625, 10.500, 10.125.
Solution: When the data is in fractions and the mean value comes in fractions the following
method may be used to avoid tedious calculations.
1
δ X = ( N y − X x ) X

1
or δ M = ( N y − M x ) M

where X/M is sum of items above Mean/Median X/M is sum below Mean/
y
y
y
x
Median.
104.25
Mean for the data given = = 10.425.
10
The value of items above mean ( )
X
y
10.500 + 10.500 + 10.625 + 10.625 + 10.750 = 53.
The value of items below mean ( )
X
x
10.125 + 10.125 + 10.250 + 10.375 + 10.375 = 51.25.

1
Mean deviation = ( − )53 51.25
10
1.75
= = 0.175.
10
(This method is also called short-cut method of calculation Mean deviation).

LOVELY PROFESSIONAL UNIVERSITY 93