Page 169 - DEDU504_EDUCATIONAL_MEASUREMENT_AND_EVALUATION_ENGLISH

Unit 12 : Conversion of Raw Scores into Standard Scores, T-scores, C-scores, Z-scores, Stanine Scores, Percentiles

X = Raw score Notes
M = Mean
σ = Standard deviation
And if Z-score of the raw score is known, the following formula is used :
T-score, T = 50 + 10Z
Example 5
Look at example 4. The score in mathematics = 80 and Z-score = 2.5; and the score in language =
70 and Z-score = 3. Convert these scores into T-scores.
Calculation
T-score of mathematics raw score 80, (T) = 50 + 10Z
= 50 + 10 x 2.5
= 50 + 25
= 75
And, T-score of language raw score 70, (T) = 50 + 10Z
= 50 10 3+ ×
= 50 + 30
= 80
It is clear that the student A is more able in language as compared with mathematics.
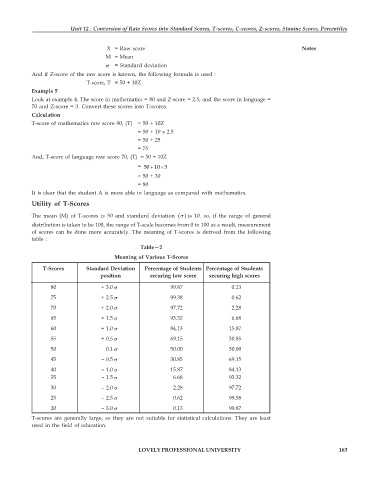
Utility of T-Scores

σ
The mean (M) of T-scores is 50 and standard deviation () is 10. so, if the range of general
distribution is taken to be 100, the range of T-scale becomes from 0 to 100 as a result, measurement
of scores can be done more accurately. The meaning of T-scores is derived from the following
table :
Table—2
Meaning of Various T-Scores

T-Scores Standard Deviation Percentage of Students Percentage of Students
position securing low score securing high scores
80 + 3.0σ 99.87 0.13

75 + 2.5σ 99.38 0.62
70 + 2.0σ 97.72 2.28
65 + 1.5σ 93.32 6.68
60 + 1.0σ 84.13 15.87
55 + 0.5σ 69.15 30.85
50 0.1σ 50.00 50.00
45 – 0.5σ 30.85 69.15

40 – 1.0σ 15.87 84.13
35 – 1.5σ 6.68 93.32
30 – 2.0σ 2.28 97.72
25 – 2.5σ 0.62 99.38

20 – 3.0σ 0.13 99.87
T-scores are generally large, so they are not suitable for statistical calculations. They are least
used in the field of education.

LOVELY PROFESSIONAL UNIVERSITY 163