Page 166 - DEDU504_EDUCATIONAL_MEASUREMENT_AND_EVALUATION_ENGLISH

Educational Measurement and Evaluation

Notes

A common way to make comparisons is to calculate z-scores. A z-score tells how
many standard deviations someone is above or below the mean. A z-score of -1.4
indicates that someone is 1.4 standard deviations below the mean. Someone who is
in that position would have done as well or better than 8% of the students who took
the test.
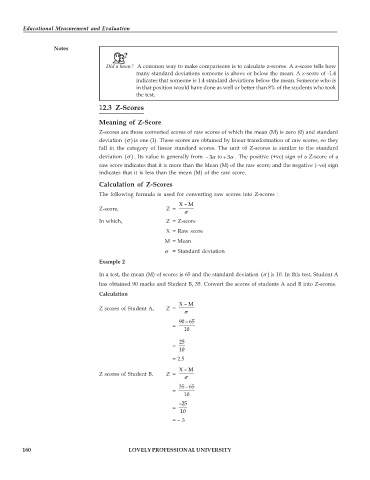
12.3 Z-Scores

Meaning of Z-Score
Z-scores are those converted scores of raw scores of which the mean (M) is zero (0) and standard
σ
deviation () is one (1). These scores are obtained by linear transformation of raw scores, so they
fall in the category of linear standard scores. The unit of Z-scores is similar to the standard
σ
deviation () . Its value is generally from –3σ to 3σ+ . The positive (+ve) sign of a Z-score of a
raw score indicates that it is more than the Mean (M) of the raw score; and the negative (–ve) sign
indicates that it is less than the mean (M) of the raw score.
Calculation of Z-Scores
The following formula is used for converting raw scores into Z-scores :

X – M
Z-score, Z =
σ
In which, Z = Z-score
X = Raw score
M = Mean
σ = Standard deviation
Example 2

In a test, the mean (M) of scores is 65 and the standard deviation () is 10. In this test, Student A
σ
has obtained 90 marks and Student B, 35. Convert the scores of students A and B into Z-scores.
Calculation
X – M
Z scores of Student A, Z =
σ
90 – 65
=
10
25
=
10
= 2.5

X – M
Z scores of Student B, Z =
σ
35 – 65
=
10
–25
=
10
= – 3

160 LOVELY PROFESSIONAL UNIVERSITY