Page 115 - DMTH401_REAL ANALYSIS

Unit 9: Functions

Notes
x  x x  x
 Either sin 1 2 = 0, or cos 1 2 = 0.
2 2
x  x x  x
If sin 1 2 = 0, then 1 2 = 0,  , + 2, ...
2 2

x  x x  x  3
If cos 1 2 = 0, then 1 2 = 0,  ,  , ...
2 2 2 2
 
Since x , x  [–  ]. Therefore we can only have
1 2
2 2
 x  x 
–  1 2 
2 2 2
 x  x 
and –  1 2 
2 2 2
x  x x  x 
Thus, 1 2 = 0 i.e., x = x . Also, if 1 2 = 
2 1 2 2 2
i.e. then x + x =  x.
1 2
x x
Since x,, x,  [– , ],
2 2
 
therefore, x = x = or x, = x = –
1 2 1
2 2
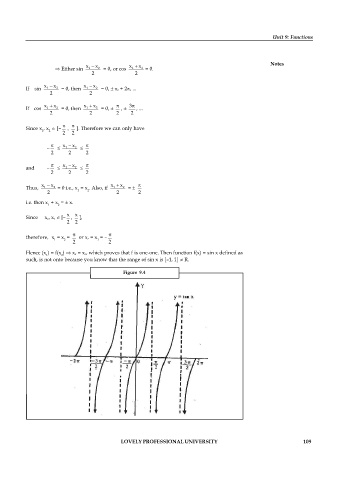
Hence (x ) = f(x )  x, = x,, which proves that f is one-one. Then function f(x) = sin x defined as
1 2
such, is not onto because you know that the range of sin x is [–1, 1]  R.
Figure 9.4

LOVELY PROFESSIONAL UNIVERSITY 109