Page 184 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 16: Tensor Fields Differentiation of Tensors

But now, apart from (7), we should have inverse formulas for (6) as well: Notes

2
1
1
3
 x = x (x , x , x );
1
 2 2 1 2 3

 x = x (x , x , x ); ...(8)
 x = x (x , x , x ).
2
3
1
3
3
 
The couple of formulas (5) and (6), and another couple of formulas (7) and (8), in the case of
tensor fields play the same role as transformation formulas in the case of free tensors.
16.2 Change of Cartesian Coordinate System
Note that formulas (6) and (8) are written in abstract form. They only indicate the functional
dependence of new coordinates of the point P from old ones and vice versa. Now we shall
specify them for the case when one Cartesian coordinate system is changed to another Cartesian
coordinate system. Remember that each Cartesian coordinate system is determined by some
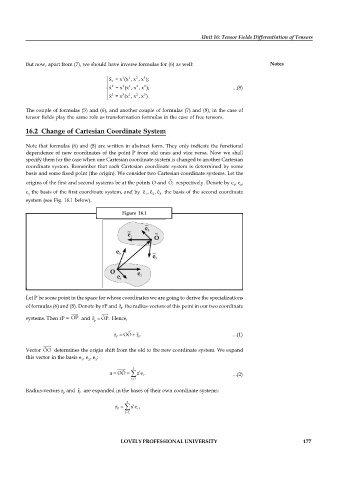
basis and some fixed point (the origin). We consider two Cartesian coordinate systems. Let the
origins of the first and second systems be at the points O and O, respectively. Denote by e , e ,

1
2
e the basis of the first coordinate system, and by e , e , e  the basis of the second coordinate


1
3
2
3
system (see Fig. 16.1 below).
Figure 16.1

Let P be some point in the space for whose coordinates we are going to derive the specializations
of formulas (6) and (8). Denote by rP and r  the radius-vectors of this point in our two coordinate
P
 


systems. Then rP = OP and r  OP. Hence,
p



r  OO r .  P ...(1)
P

Vector OO determines the origin shift from the old to the new coordinate system. We expand

this vector in the basis e , e , e :
3
1
2
 3
i

a  OO   a e . ...(2)
i
i 1

Radius-vectors r and r  are expanded in the bases of their own coordinate systems:
P
P
3
i
P 
r  x e ,
i
i 1

LOVELY PROFESSIONAL UNIVERSITY 177