Page 231 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

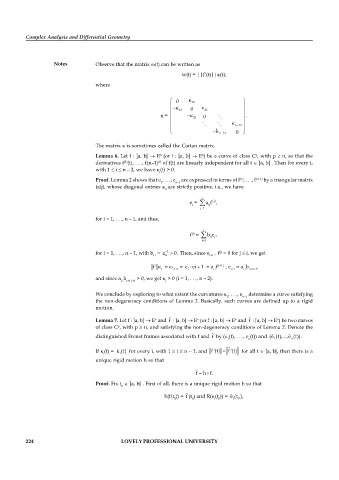
Notes Observe that the matrix (t) can be written as
w(t) = ||f’(t)||(t),

where

 0  12 
 
  12 0  23 
 =   23 0  . 
 
    n 1n 

 k 
  n 1n 0 

The matrix  is sometimes called the Cartan matrix.
Lemma 6. Let f : ]a, b[  E (or f : [a, b]  E ) be a curve of class C , with p  n, so that the
n
n
p
derivatives f (t), . . ., f(n–1) of f(t) are linearly independent for all t  ]a, b[ . Then for every i,
(1)
(t)
with 1  i  n – 2, we have  (t) > 0.
i
Proof. Lemma 2 shows that e , . . ., e are expressed in terms of f , . . ., f (n–1) by a triangular matrix
(1)
1
n–1
(aij), whose diagonal entries a are strictly positive, i.e., we have
ii
i
e = a f ,
( j)
i 
ij
j 1

for i = 1, . . ., n – 1, and thus,
i
f =  b e ,
(i)
j
ij
j 1

1

(j)
for i = 1, . . ., n – 1, with b = a  0. Then, since e . f = 0 for j  i, we get
ii
i+1
i i
'


f'  =  i i+1 = e ei 1 = a f (i+1) . e = a b ,
i
i
i+1
i i
i i i+1i+1
and since a b > 0, we get  > 0 (i = 1, . . ., n – 2).
i i i+1 i+1
i
More on Frenet . . .
We conclude by exploring to what extent the curvatures  , . . .,  determine a curve satisfying
n–1
1
the non-degeneracy conditions of Lemma 2. Basically, such curves are defined up to a rigid
motion.

Lemma 7. Let f : ]a, b[  E and f : ]a, b[  E (or f : [a, b]  E and f : [a, b]  E ) be two curves

n
n
n
n
of class C , with p  n, and satisfying the non-degeneracy conditions of Lemma 2. Denote the
p
distinguished Frenet frames associated with f and f by (e (t), . . ., e (t)) and (e (t),...,e (t)).



n
1
1
n
If  (t) =   i (t) for every i, with 1  i  n – 1, and f'(t)   f'(t) for all t  ]a, b[, then there is a
i
unique rigid motion h so that
 f  h f.

Proof. Fix t  ]a, b[ . First of all, there is a unique rigid motion h so that
0
h(f(t )) = f (t ) and R(e (t )) = e (t ),


i
0
0
0
0
i
224 LOVELY PROFESSIONAL UNIVERSITY