Page 29 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes 3.1 The Exponential Function

Let the so-called exponential function exp be defined by

exp(z) = ex(cos y + i sin y),
where, as usual, z = x + iy. From the Cauchy-Riemann equations, we see at once that this function
has a derivative every where—it is an entire function. Moreover,

d exp(z).
dz exp(z) 
Note next that if z = x + iy and w = u + iv, then
exp(z + w) = e [cos(y + v) + i sin(y + v)]
x+u
= e e [cos y cos v – sin y sin v + i(sin y cos v + cos y sin v)]
x u
= e e (cos y + i sin y) (cos v + i sin v)
x u
= exp(z) exp(w).
We, thus, use the quite reasonable notation e = exp(z) and observe that we have extended the
z
real exponential e to the complex numbers.
x

Example: Recall from elementary circuit analysis that the relation between the voltage
drop V and the current flow I through a resistor is V = RI, where R is the resistance. For an
dl dV
inductor, the relation is V = L , where L is the inductance; and for a capacitor, C = I, where
dt dt
C is the capacitance. (The variable t is, of course, time.) Note that if V is sinusoidal with a
frequency , then so also is I. Suppose then that V = A sin(t + ). We can write this as
V = Im(Ae e ) = Im(Be ), where B is complex. We know the current I will have this same form:
it
i it
I = Im (Ce ). The relations between the voltage and the current are linear, and so we can consider
it
complex voltages and currents and use the fact that e = cos t + i sin t. We, thus, assume a more
it
or less fictional complex voltage V, the imaginary part of which is the actual voltage, and then
the actual current will be the imaginary part of the resulting complex current.
What makes this a good idea is the fact that differentiation with respect to time t becomes simply
d
multiplication by i: Ae = iwtAe . If I = be , the above relations between current and
it
it
it
dt
1
voltage become V = iLI for an inductor, and iVC = I, or V = for a capacitor. Calculus is
 i C
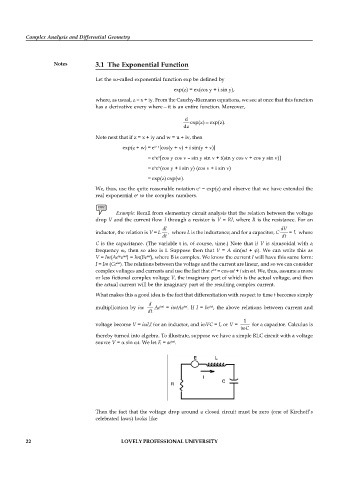
thereby turned into algebra. To illustrate, suppose we have a simple RLC circuit with a voltage
source V =  sin t. We let E = ae .
it

Then the fact that the voltage drop around a closed circuit must be zero (one of Kirchoff’s
celebrated laws) looks like

22 LOVELY PROFESSIONAL UNIVERSITY