Page 30 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 3: Elementary Functions

I Notes
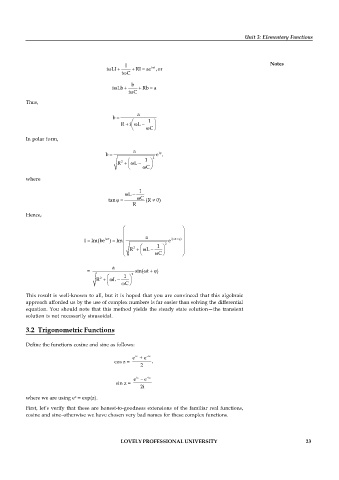
i t

i LI   RI  ae ,or

i C

b
i Lb   Rb  a

i C

Thus,
a
b 
i  
R    L 1  
  C 
In polar form,
a
i
b  2 e ,

L
2
R    1  

  C 
where
1
 L 
tan   C (R  0)
R
Hence,
 
 a 
i t
)

I  Im(be ) Im   2 e i( t  



L
2
 R    1  
   
   C  
a
= 2 sin( t   )


2
L
R    1  

  C 
This result is well-known to all, but it is hoped that you are convinced that this algebraic
approach afforded us by the use of complex numbers is far easier than solving the differential
equation. You should note that this method yields the steady state solution—the transient
solution is not necessarily sinusoidal.
3.2 Trigonometric Functions
Define the functions cosine and sine as follows:
e + e –iz
iz
cos z = ,
2
iz
e – e –iz
sin z =
2i
where we are using e = exp(z).
z
First, let’s verify that these are honest-to-goodness extensions of the familiar real functions,
cosine and sine–otherwise we have chosen very bad names for these complex functions.

LOVELY PROFESSIONAL UNIVERSITY 23