Page 409 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes which says that points P(x , y ) and P(x , y ) lie on the straight line
2 2 3 3
s = 0. ...(20)
1
The latter is uniquely determined by P(x , y ), which, too, can be retrieved from (20). We define
1
1
s = 0 as the polar of P(x , y ) with respect to the conic s = 0. P(x , y ) is said to be the pole of its
1
1
1
1
1
polar. Obviously, for a point on the conic, the polar is exactly the tangent at this point.
Thus we see that the pole/polar definitions generalize naturally from the circle to other non-
degenerate conics. We now prove La Hire’s
Theorem 12
If point P(x , y ) lies on the polar of P(x , y ) with respect to a conic s = 0, then P(x , y ) lies on the
1
2
2
2
1
2
polar of P(x , y ) with respect to the same conic.
1
1
Proof
Indeed, P(x , y ) lies on the polar s = 0 if and only if s = 0. Because of the symmetry of the
1
2
1
21
notations, this is the same as s = 0, which says that P(x , y ) lies on s = 0.
12
2
1
2
31.4 Tissot’s Theorem
At any point on a reference globe there are an infinite number of paired orthogonal directions.
When transformed to map they may or may not remain orthogonal
Tissot’s theorem states that regardless of the type of transformation, at each point on a sphere
there is at least one pair of orthogonal directions that will remain orthogonal when transformed
Referred to as principle directions; a and b and it is not important what directions actually are
Tissot’s theory of distortions states that
A circle on the datum surface with a centre P and a radius ds may be assumed to be a plane figure
within its infinitely small area. This area will remain infinitely small and plane on the projection
surface. Generally the circle will be portrayed as a ellipse.
This ellipse is called Tissot’s Indicatrix as it indicates the characteristics of a projection in the direct
environment of a point.
The axes of Tissot’s Indicatrix correspond to the two principal directions and the maximum and
minimum particular scales, a and b, at any point, occur in these directions.
Proof That The Projected Circle Is An Ellipse

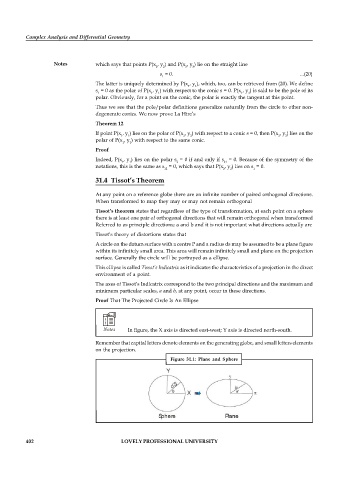
Notes In figure, the X axis is directed east-west; Y axis is directed north-south.
Remember that capital letters denote elements on the generating globe, and small letters elements
on the projection.
Figure 31.1: Plane and Sphere

402 LOVELY PROFESSIONAL UNIVERSITY