Page 411 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Complex Analysis and Differential Geometry

Notes ds
Remember from previous section: m = , so:
dS


 ds   cos '
a    
 dS  cos

 ds   sin '
b    
 dS y  sin
or
a cos  =  cos ’
b sin  =  sin ’
a cos  =  cos ’
2
2
2
2
b sin  =  sin ’
2
2
2
2
a cos  + b sin  =  (cos ’ + sin ’)
2
2
2
2
2
2
2
2
2
2
2
2
 = a cos  + b sin 
This formula expresses the length distortion in any direction as a function of the original direction
, and the principal scale factors, a and b. The angle  indicates the direction of the parallel with
respect to the x axis. The direction of the meridian with respect to the x axis is thus
 + 90º =  + /2 = 
The scale distortions along the parallels and meridians (note: not necessarily equal to the
maximum and minimum distortions along a and b) are thus:
2
2
2
2
2
 = a sin  + b cos 

2
2
2
2
 2  = a sin  + b cos  = a cos  + b sin 
2
2
2
2
2
2
  m = a + b 2
2


This is known as the First Theorem of Appolonius:
The sum of the squares of the two conjugate diameters of an ellipse is constant.
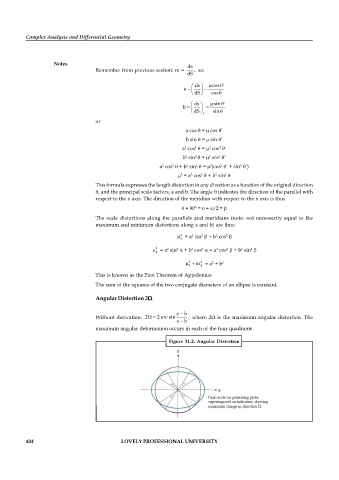
Angular Distortion 2
a b

Without derivation: 2  2 arc sin , where 2 is the maximum angular distortion. The
a b

maximum angular deformation occurs in each of the four quadrants.
Figure 31.2: Angular Distortion

404 LOVELY PROFESSIONAL UNIVERSITY