Page 80 - DMTH402_COMPLEX_ANALYSIS_AND_DIFFERENTIAL_GEOMETRY

Unit 7: Transformations and Conformal Mappings

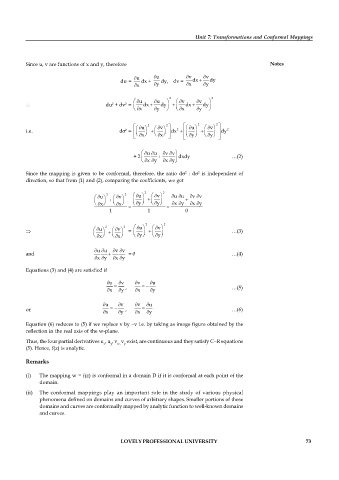
Since u, v are functions of x and y, therefore Notes

du =  u dx +  u dy, dv =  v dx   v dy
 x  y  x  y

  u  u  2   v  v  2
2
2
 du + dv =    x dx   y dy       x dx   y dy  
  u 2   v 2     u 2   v 2 

2
i.e. d =       dx         dy 2
2
   x    x       y   y  
v v

+ 2    u u     dxdy (2)

x y

x y



Since the mapping is given to be conformal, therefore, the ratio d : d is independent of
2
2
direction, so that from (1) and (2), comparing the coefficients, we get


  u 2   v 2   u 2    v 2  u u  v v
         


  x    x     y   y   x y  x y
1 1 0
2 v 2
u
2
2


     u       v  =    y      y  (3)



x 
x 



and  u u   v v = 0 (4)
 x y  x y


Equations (3) and (4) are satisfied if
 u   v  v    u
 x  y ,  x  y (5)
 u  v  v  u
or  x    y ,  x   y (6)
Equation (6) reduces to (5) if we replace v by v i.e. by taking as image figure obtained by the
reflection in the real axis of the w-plane.
Thus, the four partial derivatives u , u , v v exist, are continuous and they satisfy CR equations
y
x
x­, y
(5). Hence, f(z) is analytic.
Remarks
(i) The mapping w = f(z) is conformal in a domain D if it is conformal at each point of the
domain.
(ii) The conformal mappings play an important role in the study of various physical
phenomena defined on domains and curves of arbitrary shapes. Smaller portions of these
domains and curves are conformally mapped by analytic function to well-known domains
and curves.

LOVELY PROFESSIONAL UNIVERSITY 73