Page 232 - DMTH404_STATISTICS

Statistics

Notes Even if e  e , we can assume that V = V Then: V = V  V  V  V  V  V
1 2 e 1 e 2 x 1 t e 1 t e 2 x 2 x
 (x * x )  [(t e )(t e )]


C  1 2  1 2 
x 1 x 2 N N
C x 1 x 2  V  Ct e 1  Ct e 2  C e 1 2 e  V t
t
C C V
r  x 1 x 2  x 1 2 x  t
x 1 x 2
V * V 2 x V x V x
x
1
V
r  t  rxt 2
x 1 x 2 V
x
The reliability is the correlation between two parallel tests and is equal to the squared correlation
of the test with the construct.
V t
r = = percent of test variance which is construct variance. rxt = rxx  the validity of a test
xx V x
is bounded by the square root of the reliability.
How do we tell if one of the two “parallel” tests is not as good as the other? That is, what if the
two tests are not parallel?
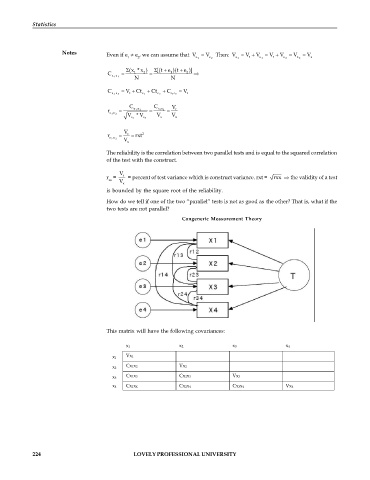
Congeneric Measurement Theory

This matrix will have the following covariances:

x1 x2 x3 x4
x1 Vx1
x2 Cx1x2 Vx2
x3 Cx1x3 Cx2x3 Vx3
x4 Cx1x4 Cx2x4 Cx3x4 Vx4

224 LOVELY PROFESSIONAL UNIVERSITY