Page 237 - DMTH404_STATISTICS

Unit 16: Reliability Theory

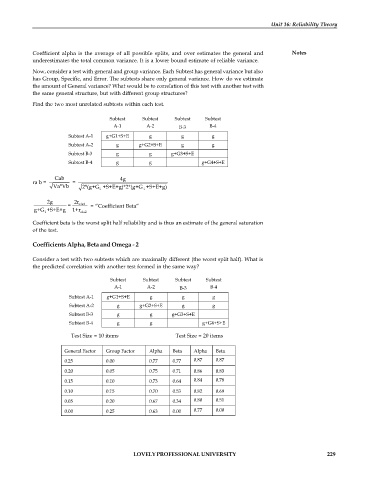
Coefficient alpha is the average of all possible splits, and over estimates the general and Notes
underestimates the total common variance. It is a lower bound estimate of reliable variance.
Now, consider a test with general and group variance. Each Subtest has general variance but also
has Group, Specific, and Error. The subtests share only general variance. How do we estimate
the amount of General variance? What would be to correlation of this test with another test with
the same general structure, but with different group structures?
Find the two most unrelated subtests within each test.

Subtest Subtest Subtest Subtest
A-1 A-2 B-3 B-4
Subtest A-1 g+G1+S+E g g g
Subtest A-2 g g+G2+S+E g g
Subtest B-3 g g g+G3+S+E
Subtest B-4 g g g+G4+S+E

Cab 4g
ra b = =
Va*Vb 2*(g+G +S+E+g)*2*(g+G +S+E+g)
i i
2g 2r
= a1a2 = “Coefficient Beta”
g+G +S+E+g 1+r a1a2
1
Coefficient beta is the worst split half reliability and is thus an estimate of the general saturation
of the test.

Coefficients Alpha, Beta and Omega - 2

Consider a test with two subtests which are maximally different (the worst split half). What is
the predicted correlation with another test formed in the same way?

Subtest Subtest Subtest Subtest
A-1 A-2 B-3 B-4
Subtest A-1 g+G1+S+E g g g
Subtest A-2 g g+G2+S+E g g
Subtest B-3 g g g+G3+S+E
Subtest B-4 g g g+G4+S+E

Test Size = 10 items Test Size = 20 items

General Factor Group Factor Alpha Beta Alpha Beta
0.25 0.00 0.77 0.77 0.87 0.87
0.20 0.05 0.75 0.71 0.86 0.83
0.15 0.10 0.73 0.64 0.84 0.78
0.10 0.15 0.70 0.53 0.82 0.69
0.05 0.20 0.67 0.34 0.80 0.51
0.00 0.25 0.63 0.00 0.77 0.00

LOVELY PROFESSIONAL UNIVERSITY 229