Page 235 - DMTH404_STATISTICS

Unit 16: Reliability Theory

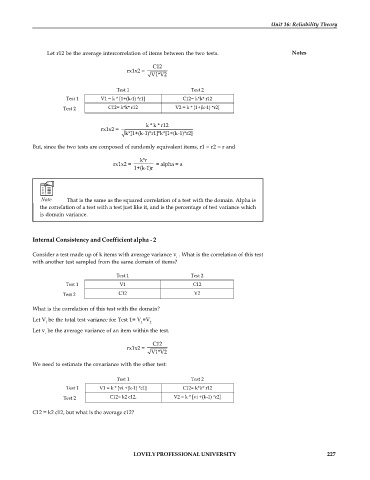
Let r12 be the average intercorrelation of items between the two tests. Notes

C12
rx1x2 =
V1*V2

Test 1 Test 2
Test 1 V1 = k * [1+(k-1) *r1] C12= k*k* r12
Test 2 C12= k*k* r12 V2 = k * [1+(k-1) *r2]

k * k * r12
rx1x2 =
k*[1+(k-1)*r1]*k*[1+(k-1)*r2]

But, since the two tests are composed of randomly equivalent items, r1 = r2 = r and
k*r
rx1x2 = = alpha = a
1+(k-1)r

Note That is the same as the squared correlation of a test with the domain. Alpha is
the correlation of a test with a test just like it, and is the percentage of test variance which
is domain variance.

Internal Consistency and Coefficient alpha - 2

Consider a test made up of k items with average variance v . What is the correlation of this test
i
with another test sampled from the same domain of items?
Test 1 Test 2
Test 1 V1 C12
Test 2 C12 V2

What is the correlation of this test with the domain?

Let V be the total test variance for Test 1= V =V
t 1 2
Let v be the average variance of an item within the test.
i
C12
rx1x2 =
V1*V2

We need to estimate the covariance with the other test:

Test 1 Test 2
Test 1 V1 = k * [vi +(k-1) *c1] C12= k*k* r12
Test 2 C12= k2 c12, V2 = k * [vi +(k-1) *c2]

C12 = k2 c12, but what is the average c12?

LOVELY PROFESSIONAL UNIVERSITY 227