Page 337 - DMTH404_STATISTICS

Unit 23: Regression Analysis

23.2 Regression Coefficient in a Bivariate Frequency Distribution Notes

As in case of calculation of correlation coefficient (see § 12.6), we can directly write the formula
for the two regression coefficients for a bivariate frequency distribution as given below :

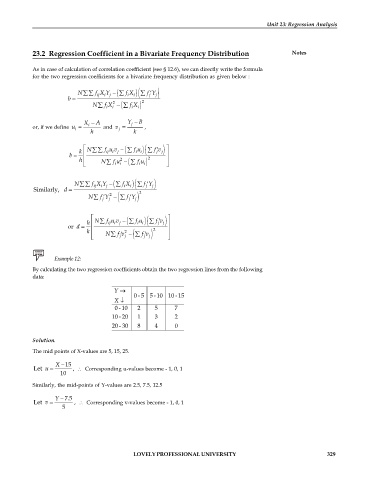
Nåå f X Y - (å f X i )(å f Y ¢ j j )
i
i j
ij
b = 2
2
Nå f X - (å f X )
i i i i
X - A Y - B
j
i
or, if we define u = and v = ,
i
j
h k
é ) ù
k Nåå f u v - (å f u )(å f v ¢
j j
ij i j
i i
b = ê ú
h ê Nå f u - (å f u ) 2 ú
2
ë i i i i û
Nåå f X Y - (å f X i )(å f Y ¢ j j )
ij
i
i j
Similarly, d =
2 2
¢
Nå f Y - (å f Y ¢ j j )
j
j
é ) ù
ij i j
h ê Nå f u v - (å f u )(å f v ¢ j j ú
i i
or d = 2
k ê 2 ) ú
¢
ê Nå f v - (å f v ¢ j j ú
j j
ë û
Example 12:
By calculating the two regression coefficients obtain the two regression lines from the following
data:
Y 
XB 0 - 5 5 -10 10 -15
0 -10 2 5 7
10 - 20 1 3 2
20 - 30 8 4 0
Solution.
The mid points of X-values are 5, 15, 25.

X 15
-
Let u = ,  Corresponding u-values become - 1, 0, 1
10
Similarly, the mid-points of Y-values are 2.5, 7.5, 12.5

-
Y 7.5
Let v = ,  Corresponding v-values become - 1, 0, 1
5

LOVELY PROFESSIONAL UNIVERSITY 329