Page 338 - DMTH404_STATISTICS

Statistics

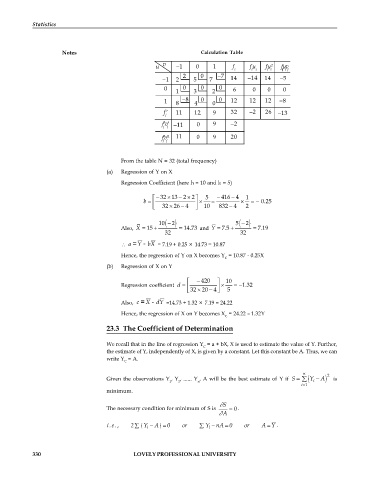
Notes Calculation Table

v 2
u –1 0 1 f f u fu fuv
i i i i i ij i j
2 0 –7
–1 2 5 7 14 –14 14 –5
0 0 0 0 6 0 0 0
1 3 2
1 8 –8 4 0 0 0 12 12 12 –8

f¢ 11 12 9 32 –2 26 –13
j
f¢v¢ –11 0 9 –2
j j
f¢v¢ 2 11 0 9 20
j j

From the table N = 32 (total frequency)
(a) Regression of Y on X
Regression Coefficient (here h = 10 and k = 5)

´
-
´
-
é - 32 13 2 2 ù 5 - 416 4 1
b = ´ = ´ = - 0.25
ê ú
´
-
-
ë 32 26 4 û 10 832 4 2
10 ( ) 2- 5 ( ) 2-
Also, X = 15 + = 14.73 and Y = 7.5 + = 7.19
32 32

 a =Y - bX = 7.19 + 0.25 ´ 14.73 = 10.87
Hence, the regression of Y on X becomes Y = 10.87 - 0.25X
C
(b) Regression of X on Y
é - 420 ù 10
Regression coefficient d = ´ = - 1.32
ê ú
´
-
ë 32 20 4 û 5
Also, c = X - dY =14.73 + 1.32 ´ 7.19 = 24.22
Hence, the regression of X on Y becomes X = 24.22 – 1.32Y
C
23.3 The Coefficient of Determination
We recall that in the line of regression Y = a + bX, X is used to estimate the value of Y. Further,
C
the estimate of Y, independently of X, is given by a constant. Let this constant be A. Thus, we can
write Y = A.
C
n 2
Y -
Given the observations Y , Y , ...... Y , A will be the best estimate of Y if S = å ( i A ) is
1 2 n
=
i 1
minimum.
¶ S
The necessary condition for minimum of S is = 0 .
¶ A
=
i.e., 2 å ( Y i - A ) = 0 or å Y i - nA 0 or A Y .
=

330 LOVELY PROFESSIONAL UNIVERSITY