Page 353 - DMTH404_STATISTICS

Unit 24: Sampling Distributions

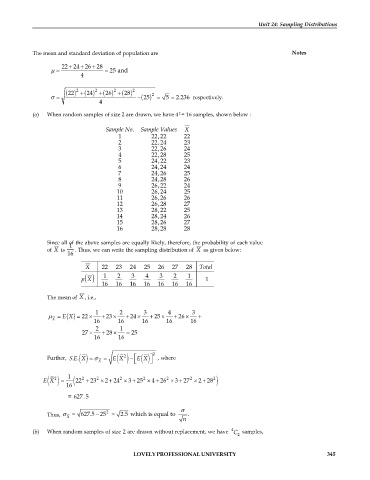
The mean and standard deviation of population are Notes

+
+
+
22 24 26 28
m = = 25 and
4
2 2 2 2
26
24
28
22
( ) + ( ) + ( ) + ( ) 2
25
s = - ( ) = 5 = 2.236 respectively.
4
(a) When random samples of size 2 are drawn, we have 4 = 16 samples, shown below :
2
Sample No. Sample Values X
1 22,22 22
2 22,24 23
3 22,26 24
4 22,28 25
5 24,22 23
6 24,24 24
7 24,26 25
8 24,28 26
9 26,22 24
10 26,24 25
11 26,26 26
12 26,28 27
13 28,22 25
14 28,24 26
15 28,26 27
16 28,28 28

Since all of the above samples are equally likely, therefore, the probability of each value
1
of X is . Thus, we can write the sampling distribution of X as given below:
16
X 22 23 24 25 26 27 28 Total
p X d i 1 2 3 4 3 2 1 1
16 16 16 16 16 16 16

The mean of X , i.e.,

1 2 3 4 3
( ) =
m = E X 22 ´ + 23 ´ + 24 ´ + 25 ´ + 26 ´ +
X
16 16 16 16 16
2 1
27 ´ + 28 ´ = 25
16 16
2
( )
Further, S E X. . ( ) s= X = E X 2 é ë E X ù , where
( ) -
û
1
2 2 2 2 2 2 2 2
+
E X
( ) = ( 22 + 23 ´ 2 24 ´ 3 25 ´ 4 26 ´ 3 27 ´ 2 28 )
+
+
+
+
16
= 627.5
s
2
-
Thus, s = 627.5 25 = 2.5 which is equal to .
X
n
4
(b) When random samples of size 2 are drawn without replacement, we have C samples,
2
LOVELY PROFESSIONAL UNIVERSITY 345