Page 64 - DMTH404_STATISTICS

Statistics

Notes
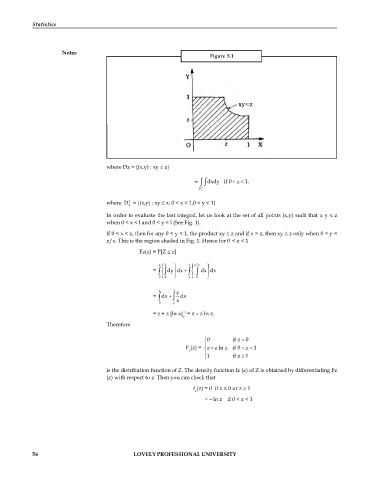
Figure 5.1

where Dz = {(x,y) : xy  z}


   dxdy if 0 z 1.

1
D
z
1
where D = {(x,y) : xy  z, 0 < x < 1,0 < y < 1}
z
In order to evaluate the last integral, let us look at the set of all points (x,y) such that x y  z
when 0 < x < l and 0 < y < l (See Fig. 1).
If 0 < x < z, then for any 0 < y < 1, the product xy  z and if x > z, then xy  z only when 0 < y <
z/x. This is the region shaded in Fig. 1. Hence for 0 < z < 1

Fz(z) = P[Z  z]
z 1 1 z /x
       
=    dy dx     dx dx


0   0   z   0  
z 1 z

= dx   dx
0 z x
1
= z + z [ln x] = z – z ln z.
z
Therefore

 0 if z  0

F (z) = z z ln z if 0  z  1

z

 1 if z  1
is the distribution function of Z. The density function fz (z) of Z is obtained by differentiating Fz
(z) with respect to z. Then you can check that
f (z) = 0 if z  0 or z  1
z
= – ln z if 0 < z < 1

56 LOVELY PROFESSIONAL UNIVERSITY