Page 65 - DMTH404_STATISTICS

Unit 5: Functions of Random Variables

Notes
Example 2: Suppose X and Y are independent exponential random variables with the
density function
y
-x
f (x) = e , x > 0 and f(y) = e , y > 0
= 0 , x  0. = 0, y  0
Define Z = X + Y and let us find the distribution function of Z.

From the definition of Z,
Fz(z) = P[Z  z] = 0 if z < 0
and, for z > 0

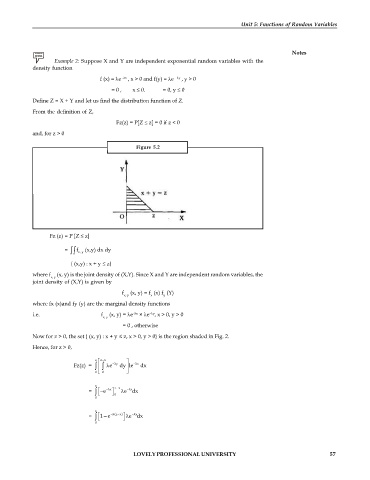
Figure 5.2

Fz (z) = P [Z  z]

=  f x, y (x,y) dx dy

{ (x,y) : x + y  z}
where f (x, y) is the joint density of (X,Y). Since X and Y are independent random variables, the
x, y
joint density of (X,Y) is given by
f (x, y) = f (x) f (Y)
x, y x y
where fx (x)and fy (y) are the marginal density functions
i.e. f (x, y) = e × e , x > 0, y > 0
–x
y
x, y
= 0 , otherwise
Now for z > 0, the set { (x, y) : x + y  z, x > 0, y > 0} is the region shaded in Fig. 2.
Hence, for z > 0,

z  z x 


Fz(z) =     e  y dy le – x dx

0  0 
z z x


 
=  e  x  0   e – x dx
0
z



=    1 e  (z x)  e – x dx

0
LOVELY PROFESSIONAL UNIVERSITY 57