Page 108 - DMTH503_TOPOLOGY

Topology

Notes 10.1.2 Quotient Topology

If X is a space and A is a set and if p : X  A is a surjective map, than there exists exactly one
topology T on A relative to which p is a quotient map; it is called the quotient topology induced
by p.
The topology T is of course defined by letting it consists of those subsets U of A such that p (U)
–1
–1
is open in X. It is easy to check that T is a topology. The sets  and A are open because p () = 
–1
and p (A) = X. The other two conditions follow from the equations
 
1

p  U   p  1 U ,
   
  J   J

1
p  1   n U i    n p (U )
i
 i 1  i 1


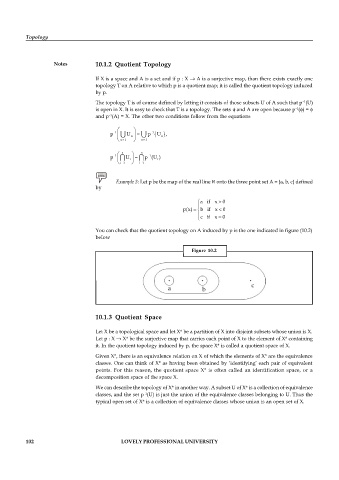
Example 3: Let p be the map of the real line onto the three point set A = {a, b, c} defined
by
 a if x  0

p(x)   b if x  0
 c if x  0

You can check that the quotient topology on A induced by p is the one indicated in figure (10.2)
below
Figure 10.2

10.1.3 Quotient Space

Let X be a topological space and let X* be a partition of X into disjoint subsets whose union is X.
Let p : X  X* be the surjective map that carries each point of X to the element of X* containing
it. In the quotient topology induced by p, the space X* is called a quotient space of X.

Given X*, there is an equivalence relation on X of which the elements of X* are the equivalence
classes. One can think of X* as having been obtained by ‘identifying’ each pair of equivalent
points. For this reason, the quotient space X* is often called an identification space, or a
decomposition space of the space X.
We can describe the topology of X* in another way. A subset U of X* is a collection of equivalence
classes, and the set p (U) is just the union of the equivalence classes belonging to U. Thus the
–1
typical open set of X* is a collection of equivalence classes whose union is an open set of X.

102 LOVELY PROFESSIONAL UNIVERSITY