Page 106 - DMTH503_TOPOLOGY

Topology Richa Nandra, Lovely Professional University

Notes Unit 10: The Quotient Topology

CONTENTS
Objectives
Introduction

10.1 The Quotient Topology
10.1.1 Quotient Map, Open and Closed Map
10.1.2 Quotient Topology

10.1.3 Quotient Space
10.2 Summary
10.3 Keywords
10.4 Review Questions
10.5 Further Readings

Objectives

After studying this unit, you will be able to:
 Understand the quotient map, open map and closed map;

 Explain the quotient topology;
 Solve the theorems and questions on quotient topology.
Introduction

The quotient topology is not a natural generalization of something. You have already studied in
analysis. Nevertheless, it is easy enough to motivate. One motivation comes from geometry,
where one often has occasion to use ‘cut-and-paste’ techniques to construct such geometric
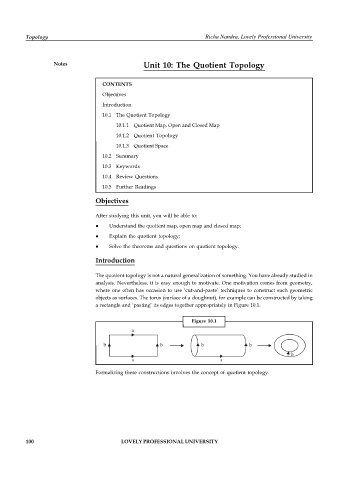
objects as surfaces. The torus (surface of a doughnut), for example can be constructed by taking
a rectangle and ‘pasting’ its edges together appropriately in Figure 10.1.

Figure 10.1

Formalizing these constructions involves the concept of quotient topology.

100 LOVELY PROFESSIONAL UNIVERSITY