Page 233 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

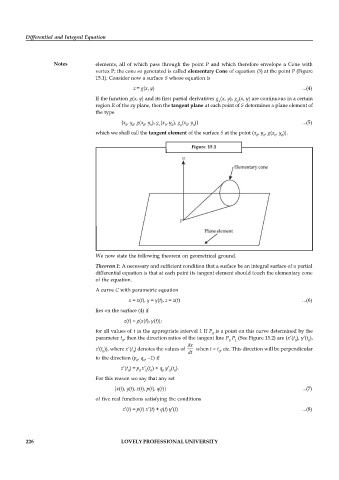
Notes elements, all of which pass through the point P and which therefore envelope a Cone with
vertex P; the cone so generated is called elementary Cone of equation (3) at the point P (Figure
15.1). Consider now a surface S whose equation is
z = g(x, y) ...(4)
If the function g(x, y) and its first partial derivatives g (x, y), g (x, y) are continuous in a certain
x y
region R of the xy plane, then the tangent plane at each point of S determines a plane element of
the type
{x , y , g(x , y ), g (x , y ), g (x , y )} ...(5)
0 0 0 0 x 0 0 y 0 0
which we shall call the tangent element of the surface S at the point (x , y , g(x , y )).
0 0 0 0
Figure 15.1

We now state the following theorem on geometrical ground.
Theorem 1: A necessary and sufficient condition that a surface be an integral surface of a partial
differential equation is that at each point its tangent element should touch the elementary cone
of the equation.
A curve C with parametric equation
x = x(t), y = y(t), z = z(t) ...(6)

lies on the surface (4) if
z(t) = g(x(t), y(t));
for all values of t in the appropriate interval l. If P is a point on this curve determined by the
0
parameter t , then the direction ratios of the tangent line P P (See Figure 15.2) are (x (t ), y (t ),
0 0 1 0 0
dx
z (t )), where x (t ) denotes the values of when t = t , etc. This direction will be perpendicular
0 0 dt 0
to the direction (p , q , 1) if
0 0
z (t ) = p x (t ) + q y (t ).
0 0 0 0 0 0 0
For this reason we say that any set
{x(t), y(t), z(t), p(t), q(t)} ...(7)
of five real functions satisfying the conditions
z (t) = p(t) x (t) + q(t) y (t) ...(8)

226 LOVELY PROFESSIONAL UNIVERSITY