Page 247 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes
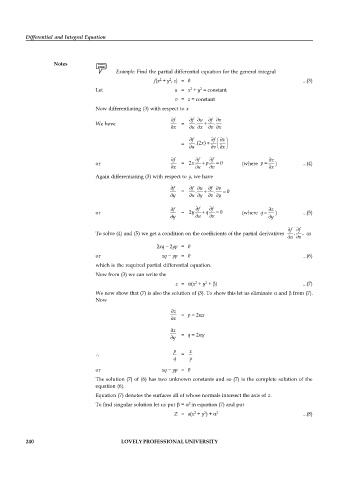
Example: Find the partial differential equation for the general integral
2
2
f(x + y , z) = 0 ...(3)
2
2
Let u = x + y = constant
v = z = constant
Now differentiating (3) with respect to x
f f u f v
We have = . .
x u x v x
f f z
x
= .(2 )
u v x
f f f z
or = 2x p 0 (where p ) ...(4)
x u v x
Again differentiating (3) with respect to y, we have

f f u f v
= . . 0
y u y v y
f f f z
or = 2y q 0 (where q ) ...(5)
y u v y

f f
To solve (4) and (5) we get a condition on the coefficients of the partial derivatives , , as
u v
2xq 2yp = 0
or xq yp = 0 ...(6)
which is the required partial differential equation.
Now from (3) we can write the
2
2
z = (x + y + ) ...(7)
We now show that (7) is also the solution of (3). To show this let us eliminate and from (7).
Now
z
= p = 2 x
x
z
= q = 2 y
y
p x
=
q y

or xq yp = 0
The solution (7) of (6) has two unknown constants and so (7) is the complete solution of the
equation (6).
Equation (7) denotes the surfaces all of whose normals intersect the axis of z.
To find singular solution let us put = 2 in equation (7) and put
2
2
Z = a(x + y ) + 2 ...(8)

240 LOVELY PROFESSIONAL UNIVERSITY