Page 363 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes at the origin r = 0. so a q and f must be equal to zero. Therefore the solution (4) reduces to
,
0
x
x
= r n (a x cos(n ) b x sin(n )) c 0 ...(5)
n 1
As a first step let us assume that the temperature on the circumference of the cylinder r = a is
specified as
= F ( ) at r R

Then placing r = R in (5) we have

F ( ) = r n (a x cos(n ) b x sin(n )) c 0 ...(6)
n 1

Now c 0 , a and b are Fourier coefficients and so are given by the relations
x
x
2
1
a = F ( )cos n d
x R n
0
2
1
b = F ( )sinn d ...(7)
x n
R
0
2
1
and c = F ( ) d
0 R
0
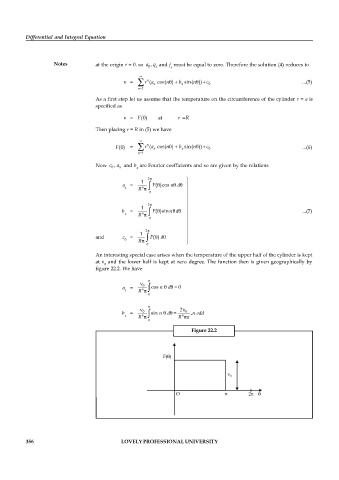
An interesting special case arises when the temperature of the upper half of the cylinder is kept
at and the lower half is kept at zero degree. The function then is given geographically by
0
figure 22.2. We have

0
a = cos n d 0
x R n
0

2
b = 0 sin n d 0 ,n odd
x n n
R R n
0
Figure 22.2

356 LOVELY PROFESSIONAL UNIVERSITY