Page 425 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

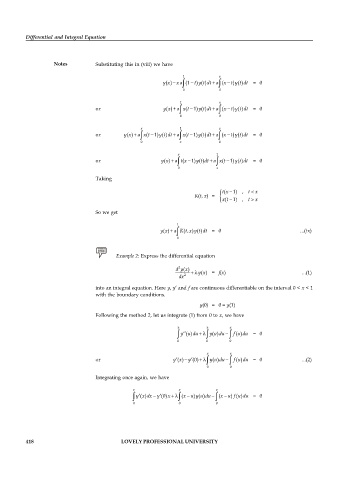
Notes Substituting this in (viii) we have
1 x
y
t
y ( ) xa (1 t ) ( )dt a (x t ) ( )dt = 0
x
t
y
0 0
1 x
or y ( ) a x (t 1) ( )dt a (x t ) ( )dt = 0
x
t
y
y
t
0 0
x 1 x
y
t
t
y
x
y
t
or y ( ) a x (t 1) ( )dt a x (t 1) ( )dt a (x t ) ( )dt = 0
0 x 0
x 1
y
t
x
y
t
or y ( ) a t (x 1) ( )dt a x (t 1) ( )dt = 0
0 x
Taking
( t x 1) , t x
K(t, x) =
( x t 1) , t x
So we get
1
x
t
y
x
y ( ) a K ( , ) ( )dt = 0 ...(ix)
t
0
Example 2: Express the differential equation
2
d y ( ) y ( ) = f(x) ...(1)
x
x
dx 2
into an integral equation. Here y, y and f are continuous differentiable on the interval 0 < x < 1
with the boundary conditions.
y(0) = 0 = y(1)
Following the method 2, let us integrate (1) from 0 to x, we have
x x x
u
u
u
y ( )du y ( )du f ( )du = 0
0 0 0
x x
x
u
or y ( ) y (0) y ( )du f ( )du = 0 ...(2)
u
0 0
Integrating once again, we have
x x x
y ( )dx y (0)x (x u ) ( )du (x u ) ( )du = 0
u
x
u
f
y
0 0 0
418 LOVELY PROFESSIONAL UNIVERSITY