Page 144 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Unit 11: Integration

Notes
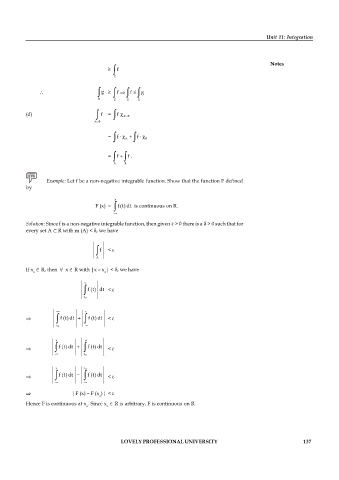
f
E

g f f g
E E E E

(d) f = f
A B
A B

= f A f B

= f f .
A B

Example: Let f be a non-negative integrable function. Show that the function F defined
by
x
F (x) = f(t) dt is continuous on R.

Solution: Since f is a non-negative integrable function, then given > 0 there is a > 0 such that for
every set A R with m (A) < , we have

f <
A

If x R, then x R with |x – x | < , we have
o o
x
f (t) dt <
x o

x
f (t) dt f (t) dt <
x o

x
f (t) dt f (t) dt <
x o
x x o
f (t) dt f (t) dt <

|F (x) – F (x )| <
o
Hence F is continuous at x . Since x R is arbitrary, F is continuous on R.
o o

LOVELY PROFESSIONAL UNIVERSITY 137