Page 250 - DMGT209_QUANTITATIVE_TECHNIQUES_II

Unit 13: Test of Significance

13.1.2 Snedecor’s F-distribution Notes

Let there be two independent random samples of sizes n1 and n2 from two normal
1 2
2
populations with variances  and  respectively. Further, let s    X  X  1 and
2
2
1
1i
1 2 n  1
1
1 2
2
s    X  X  be the variances of the first sample and the second samples respectively.
2 2i 2
n  1
2
Then F - statistic is defined as the ratio of two c - variates. Thus, we can write
2
 2 n 1 1 n  1 s 2 s 2 1
1
 1
1
n  1  2  / n   1  2
F  1  1  1
 2 n 2 1 n  1 s 2  1 s 2 2
 2
2
2
n  1  2  / n   2
2 2 2
Features of F-distribution
1. This distribution has two parameters n (= n - 1) and n (= n - 1).
1 1 2 2
 2
2. The mean of F - variate with n and n degrees of freedom is and standard error is
1 2   2
2
    2      2
2 1 2
  .
   2  1    4
2
2
Notes We note that the mean will exist if  > 2 and standard error will exist if  > 4.
2 2
Further, the mean > 1.
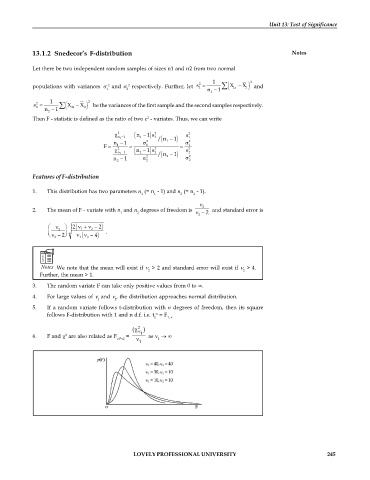
3. The random variate F can take only positive values from 0 to .
4. For large values of  and  , the distribution approaches normal distribution.
1 2
5. If a random variate follows t-distribution with n degrees of freedom, then its square
n
follows F-distribution with 1 and n d.f. i.e. t = F
2 1, 
(  )
 1
2
6. F and  are also related as F , = as   
1 2  2
1

LOVELY PROFESSIONAL UNIVERSITY 245