Page 252 - DMGT209_QUANTITATIVE_TECHNIQUES_II
P. 252
Unit 13: Test of Significance
Notes
0.1
=
0.4
31.62
0.1
= = 8.33
0.012
As the value of Z at 0.05 =1.64 and calculated value of Z falls in the rejection region, we reject null
hypothesis, and therefore we conclude that the sale of ‘Femina’ has increased significantly.
13.2.2 Chi-square Test
With the help of this test, we will come to know whether two or more attributes are associated
or not. How much the two attributes are related cannot be by Chi-Square test. Suppose, we have
certain number of observations classified according to two attributes. We may like to know
whether a newly introduced medicine is effective in the treatment of certain disease or not.
!
Caution One case where the distribution of the test statistic is an exact chi-square
distribution is the test that the variance of a normally-distributed population has a given
value based on a sample variance. Such a test is uncommon in practice because values of
variances to test against are seldom known exactly.
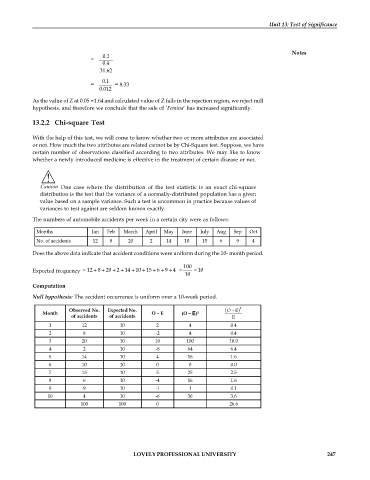
The numbers of automobile accidents per week in a certain city were as follows:
Months Jan Feb March April May June July Aug Sep Oct
No. of accidents 12 8 20 2 14 10 15 6 9 4
Does the above data indicate that accident conditions were uniform during the 10- month period.
100
Expected frequency 12 8 20 2 14 10 15 6 9 4 10
10
Computation
Null hypothesis: The accident occurrence is uniform over a 10-week period.
Observed No. Expected No. O E 2
Month O – E (O – E) 2
of accidents of accidents E
1 12 10 2 4 0.4
2 8 10 -2 4 0.4
3 20 10 10 100 10.0
4 2 10 -8 64 6.4
5 14 10 4 16 1.6
6 10 10 0 0 0.0
7 15 10 5 25 2.5
8 6 10 -4 16 1.6
9 9 10 -1 1 0.1
10 4 10 -6 36 3.6
100 100 0 26.6
LOVELY PROFESSIONAL UNIVERSITY 247