Page 186 - DCOM303_DMGT504_OPERATION_RESEARCH

Unit 9: Game Theory

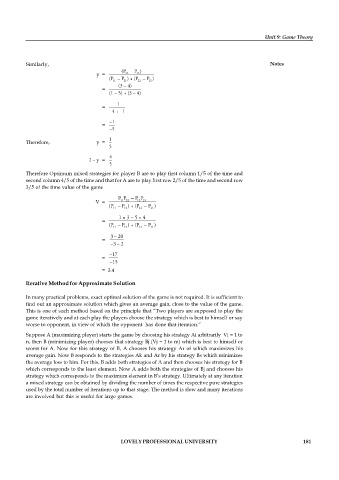
Similarly, Notes
y =

=

=

=

Therefore, y =

1 – y =

Therefore Optimum mixed strategies for player B are to play first column 1/5 of the time and
second column 4/5 of the time and that for A are to play first row 2/5 of the time and second row
3/5 of the time value of the game

V =

=


=
 

=

= 3.4

Iterative Method for Approximate Solution

In many practical problems, exact optimal solution of the game is not required. It is sufficient to
find out an approximate solution which gives an average gain, close to the value of the game.
This is one of such method based on the principle that “Two players are supposed to play the
game iteratively and at each play the players choose the strategy which is best to himself or say
worse to opponent, in view of which the opponent has done that iteration.”
Suppose A (maximizing player) starts the game by choosing his strategy Ai arbitrarily Vi = 1 to
n, then B (minimizing player) chooses that strategy Bj (Vj = 1 to m) which is best to himself or
worst for A. Now for this strategy of B, A chooses his strategy Ar of which maximizes his
average gain. Now B responds to the strategies Ak and Ar by his strategy Bs which minimizes
the average loss to him. For this, B adds both strategies of A and then chooses his strategy for B
which corresponds to the least element. Now A adds both the strategies of Bj and chooses his
strategy which corresponds to the maximum element in B’s strategy. Ultimately at any iteration
a mixed strategy can be obtained by dividing the number of times the respective pure strategies
used by the total number of iterations up to that stage. The method is slow and many iterations
are involved but this is useful for large games.

LOVELY PROFESSIONAL UNIVERSITY 181