Page 189 - DCOM303_DMGT504_OPERATION_RESEARCH

Operations Research

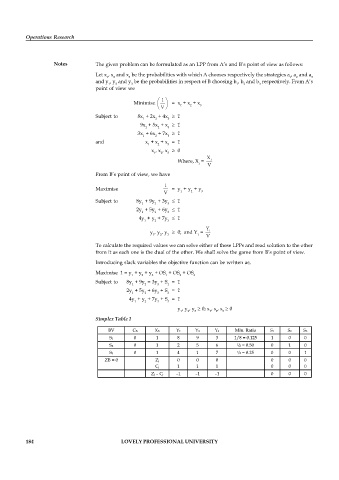
Notes The given problem can be formulated as an LPP from A’s and B’s point of view as follows:
Let x , x and x be the probabilities with which A chooses respectively the strategies a , a and a
1 2 3 1 2 3
and y , y and y be the probabilities in respect of B choosing b , b and b respectively. From A’s
1 2 3 1 2 3
point of view we
 
Minimise   = x + x + x
  1 2 3
Subject to 8x + 2x + 4x  1
1 2 3
9x + 5x + x  1
1 2 3
3x + 6x + 7x  1
1 2 3
and x + x + x = 1
1 2 3
x , x , x  0
1 2 3
Where, X =
i
From B’s point of view, we have

Maximise = y + y + y
1 2 3
Subject to 8y + 9y + 3y  1
1 2 3
2y + 5y + 6y  1
1 2 3
4y + y + 7y  1
1 2 3
y , y , y  0; and Y =
1 2 3 1
To calculate the required values we can solve either of these LPPs and read solution to the other
from it as each one is the dual of the other. We shall solve the game from B’s point of view.
Introducing slack variables the objective function can be written as,
Maximise 1 = y + y + y + OS + OS + OS
1 2 3 1 2 3
Subject to 8y + 9y = 3y + S = 1
1 2 3 1
2y + 5y + 6y + S = 1
1 2 3 2
4y + y + 7y + S = 1
1 2 3 3
y , y , y  0; s , s , s  0
1 2 3 1 2 3
Simplex Table 1
BV CB XB Y1 Y2 Y3 Min. Ratio S1 S2 S3
S1 0 1 8 9 3 1/8 = 0.125 1 0 0
S2 0 1 2 5 6 ½ = 0.50 0 1 0
S3 0 1 4 1 7 ¼ = 0.25 0 0 1
ZB = 0 Zj 0 0 0 0 0 0
Cj 1 1 1 0 0 0
Zj – Cj –1 –1 –1 0 0 0

184 LOVELY PROFESSIONAL UNIVERSITY