Page 192 - DCOM303_DMGT504_OPERATION_RESEARCH

Unit 9: Game Theory

values. The selection of strategy by player A is based on maximin principle. Similarly, the same Notes
payoff is a loss for player B. The maximum value in each column represents the maximum loss
for Player B. Player B will select the strategy that gives him the minimum loss among the
column maximum values. The selection of strategy by player B is based on minimax principle.
If the maximin value is equal to minimax value, the game has a saddle point (i.e., equilibrium
point). Thus the strategy selected by player A and player B are optimal.

Example: Consider the example to solve the game whose payoff matrix is given in
Table below:

Player B
1 2
1 1 3
Player A
2 -1 6

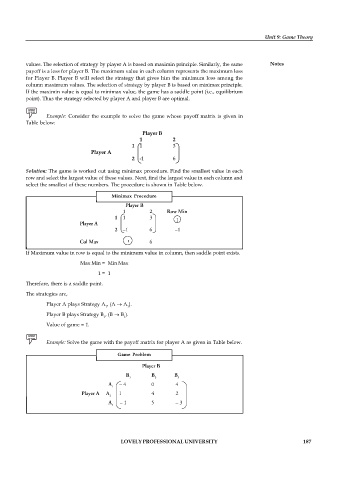
Solution: The game is worked out using minimax procedure. Find the smallest value in each
row and select the largest value of these values. Next, find the largest value in each column and
select the smallest of these numbers. The procedure is shown in Table below.

Minimax Procedure

If Maximum value in row is equal to the minimum value in column, then saddle point exists.
Max Min = Min Max

1 = 1
Therefore, there is a saddle point.
The strategies are,
Player A plays Strategy A , (A  A ).
1 1
Player B plays Strategy B , (B  B ).
1 1
Value of game = 1.

Example: Solve the game with the payoff matrix for player A as given in Table below.
Game Problem

LOVELY PROFESSIONAL UNIVERSITY 187