Page 36 - DCOM303_DMGT504_OPERATION_RESEARCH

Unit 2: Linear Programming Problems

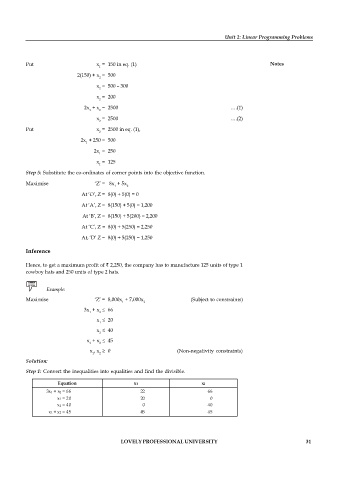
Put x = 150 in eq. (1) Notes
1
2(150) + x = 500
2
x = 500 – 300
2
x = 200
2
2x + x = 2500 ….(1)
1 2
x = 2500 ….(2)
2
Put x = 2500 in eq. (1),
2
2x + 250 = 500
1
2x = 250
1
x = 125
1
Step 5: Substitute the co-ordinates of corner points into the objective function.

Maximise ‘Z’ = 8x + 5x
1 2
At ‘O’, Z = 8(0) + 5(0) = 0
At ‘A’, Z = 8(150) + 5(0) = 1,200

At ‘B’, Z = 8(150) + 5(200) = 2,200
At ‘C’, Z = 8(0) + 5(250) = 2,250

At, ‘D’ Z = 8(0) + 5(250) = 1,250
Inference

Hence, to get a maximum profit of ` 2,250, the company has to manufacture 125 units of type 1
cowboy hats and 250 units of type 2 hats.

Example:
Maximise ‘Z’ = 8,000x + 7,000x (Subject to constraints)
1 2
3x + x  66
1 2
x  20
1
x  40
2
x + x  45
1 2
x , x  0 (Non-negativity constraints)
1 2
Solution:
Step 1: Convert the inequalities into equalities and find the divisible.

Equation x1 x2
3x 1 + x 2 = 66 22 66
x1 = 20 20 0
x 2 = 40 0 40
x1 + x2 = 45 45 45

LOVELY PROFESSIONAL UNIVERSITY 31