Page 82 - DECO405_MANAGERIAL_ECONOMICS

Unit 5: Elasticity of Demand

Notes
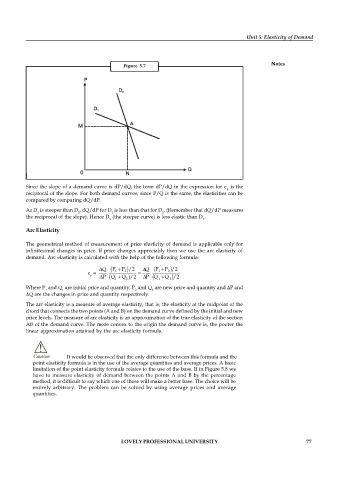
Figure 5.7

Since the slope of a demand curve is dP/dQ, the term dP/dQ in the expression for e is the
p
reciprocal of the slope. For both demand curves, since P/Q is the same, the elasticities can be
compared by comparing dQ/dP.

As D is steeper than D , dQ/dP for D is less than that for D . (Remember that dQ/dP measures
1 2 1 2
the reciprocal of the slope). Hence D (the steeper curve) is less elastic than D .
2 1
Arc Elasticity

The geometrical method of measurement of price elasticity of demand is applicable only for
infinitesimal changes in price. If price changes appreciably then we use the arc elasticity of
demand. Arc elasticity is calculated with the help of the following formula:

Q P 1 P /2 Q P 1 P /2
2
2
e = . .
p P Q Q /2 P Q Q /2
1 2 1 2
Where P and Q are initial price and quantity, P and Q are new price and quantity and P and
1 1 2 2
Q are the changes in price and quantity respectively.
The arc elasticity is a measure of average elasticity, that is, the elasticity at the midpoint of the
chord that connects the two points (A and B) on the demand curve defined by the initial and new
price levels. The measure of arc elasticity is an approximation of the true elasticity of the section
AB of the demand curve. The more convex to the origin the demand curve is, the poorer the
linear approximation attained by the arc elasticity formula.

!

Caution It would be observed that the only difference between this formula and the
point elasticity formula is in the use of the average quantities and average prices. A basic
limitation of the point elasticity formula relates to the use of the base. If in Figure 5.8 we
have to measure elasticity of demand between the points A and B by the percentage
method, it is difficult to say which one of those will make a better base. The choice will be
entirely arbitrary. The problem can be solved by using average prices and average
quantities.

LOVELY PROFESSIONAL UNIVERSITY 77