Page 187 - DMGT404 RESEARCH_METHODOLOGY

Unit 9: Correlation and Regression

Notes

Notes This formula is not applicable in case of a bivariate frequency distribution.

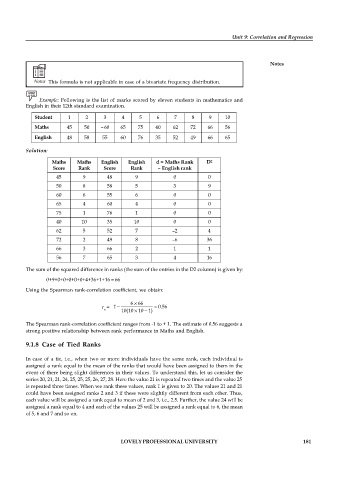
Example: Following is the list of marks scored by eleven students in mathematics and
English in their 12th standard examination.
Student 1 2 3 4 5 6 7 8 9 10
Maths 45 50 – 60 65 75 40 62 72 66 56

English 48 58 55 60 76 35 52 49 66 65

Solution:
Maths Maths English English d = Maths Rank D
2
Score Rank Score Rank – English rank
45 9 48 9 0 0
50 8 58 5 3 9
60 6 55 6 0 0
65 4 60 4 0 0
75 1 76 1 0 0
40 10 35 10 0 0
62 5 52 7 –2 4
72 2 49 8 –6 36
66 3 66 2 1 1
56 7 65 3 4 16

The sum of the squared difference in ranks (the sum of the entries in the D2 column) is given by:
0+9+0+0+0+0+0+4+36+1+16 = 66
Using the Spearman rank-correlation coefficient, we obtain:

´
6 66
r = 1 -  0.56
´
-
s 10(10 10 1)
The Spearman rank-correlation coefficient ranges from -1 to + 1. The estimate of 0.56 suggests a
strong positive relationship between rank performance in Maths and English.
9.1.8 Case of Tied Ranks

In case of a tie, i.e., when two or more individuals have the same rank, each individual is
assigned a rank equal to the mean of the ranks that would have been assigned to them in the
event of there being slight differences in their values. To understand this, let us consider the
series 20, 21, 21, 24, 25, 25, 25, 26, 27, 28. Here the value 21 is repeated two times and the value 25
is repeated three times. When we rank these values, rank 1 is given to 20. The values 21 and 21
could have been assigned ranks 2 and 3 if these were slightly different from each other. Thus,
each value will be assigned a rank equal to mean of 2 and 3, i.e., 2.5. Further, the value 24 will be
assigned a rank equal to 4 and each of the values 25 will be assigned a rank equal to 6, the mean
of 5, 6 and 7 and so on.

LOVELY PROFESSIONAL UNIVERSITY 181