Page 205 - DCOM504_SECURITY_ANALYSIS_AND_PORTFOLIO_MANAGEMENT

Security Analysis and Portfolio Management

Notes An Actual Efficient Frontier Today
This figure is an efficient frontier created from historical inputs for U.S. and international
assets over the period 1970 through 3/1995, using the Ibbotson EnCorr Optimizer program.

This is state-of-the-art portfolio selection technology. However, it is still based upon
Markowitz’s original optimization program. There are some basic features to remember:
(a) A minimum variance portfolio exists

(b) A maximum return portfolio is composed of a single asset.
(c) B, C, D & E are critical points at which the set of assets used in the frontier changes,
i.e., an asset drops out or comes in at these points.

(d) There are no assets to the northwest of the frontier. That is why we call it a frontier.
It is the edge of the feasible combinations of risk and returns.
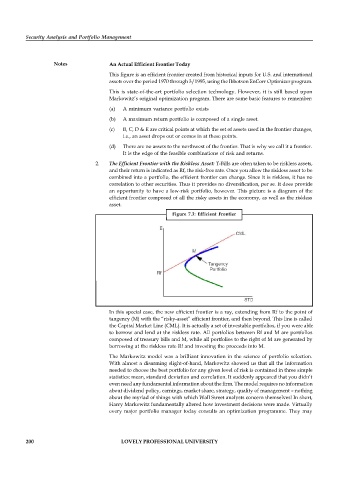
2. The Efficient Frontier with the Riskless Asset: T-Bills are often taken to be riskless assets,
and their return is indicated as Rf, the risk-free rate. Once you allow the riskless asset to be
combined into a portfolio, the efficient frontier can change. Since it is riskless, it has no
correlation to other securities. Thus it provides no diversification, per se. It does provide
an opportunity to have a low-risk portfolio, however. This picture is a diagram of the
efficient frontier composed of all the risky assets in the economy, as well as the riskless
asset.
Figure 7.3: Efficient Frontier

In this special case, the new efficient frontier is a ray, extending from Rf to the point of
tangency (M) with the “risky-asset” efficient frontier, and then beyond. This line is called
the Capital Market Line (CML). It is actually a set of investable portfolios, if you were able
to borrow and lend at the riskless rate. All portfolios between Rf and M are portfolios
composed of treasury bills and M, while all portfolios to the right of M are generated by
borrowing at the riskless rate Rf and investing the proceeds into M.

The Markowitz model was a brilliant innovation in the science of portfolio selection.
With almost a disarming slight-of-hand, Markowitz showed us that all the information
needed to choose the best portfolio for any given level of risk is contained in three simple
statistics: mean, standard deviation and correlation. It suddenly appeared that you didn’t
even need any fundamental information about the firm. The model requires no information
about dividend policy, earnings, market share, strategy, quality of management – nothing
about the myriad of things with which Wall Street analysts concern themselves! In short,
Harry Markowitz fundamentally altered how investment decisions were made. Virtually
every major portfolio manager today consults an optimization programme. They may

200 LOVELY PROFESSIONAL UNIVERSITY