Page 116 - DMTH201_Basic Mathematics-1
P. 116
Unit 4: Determinants
Notes
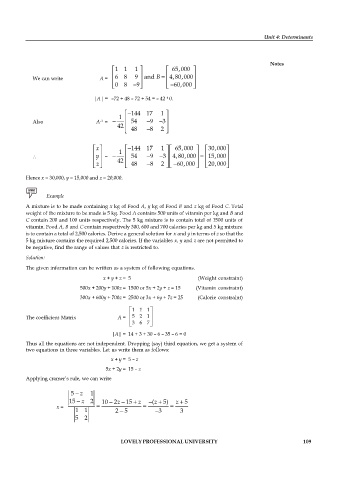
1 1 1 65,000
We can write A = 6 8 9 and B 4,80,000
0 8 9 60,000
|A| = –72 + 48 – 72 + 54 = – 42 ¹ 0.
144 17 1
1
–1
Also A = 54 9 3
42
48 8 2
x 144 17 1 65,000 30,000
1
y = 54 9 3 4,80,000 15,000
42
z 48 8 2 60,000 20,000
Hence x = 30,000, y = 15,000 and z = 20,000.
Example
A mixture is to be made containing x kg of Food A, y kg of Food B and z kg of Food C. Total
weight of the mixture to be made is 5 kg. Food A contains 500 units of vitamin per kg and B and
C contain 200 and 100 units respectively. The 5 kg mixture is to contain total of 1500 units of
vitamin. Food A, B and C contain respectively 300, 600 and 700 calories per kg and 5 kg mixture
is to contain a total of 2,500 calories. Derive a general solution for x and y in terms of z so that the
5 kg mixture contains the required 2,500 calories. If the variables x, y and z are not permitted to
be negative, find the range of values that z is restricted to.
Solution:
The given information can be written as a system of following equations.
x + y + z = 5 (Weight constraint)
500x + 200y + 100z = 1500 or 5x + 2y + z = 15 (Vitamin constraint)
300x + 600y + 700z = 2500 or 3x + 6y + 7z = 25 (Calorie constraint)
1 1 1
The coefficient Matrix A = 5 2 1
3 6 7
|A| = 14 + 3 + 30 – 6 – 35 – 6 = 0
Thus all the equations are not independent. Dropping (say) third equation, we get a system of
two equations in three variables. Let us write them as follows:
x + y = 5 – z
5x + 2y = 15 – z
Applying cramer’s rule, we can write
5 z 1
15 z 2 10 2z 15 z (z 5) z 5
x =
1 1 2 5 3 3
5 2
LOVELY PROFESSIONAL UNIVERSITY 109