Page 169 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes 1
As before interchanging y and x, we can write y x 2

To draw the graph of the two functions, we note the following points:
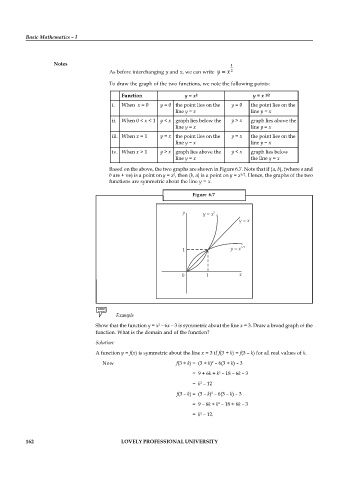
Function y = x y = x
1/2
2
i. When x = 0 y = 0 the point lies on the y = 0 the point lies on the
line y = x line y = x
ii. When 0 < x < 1 y < x graph lies below the y > x graph lies above the
line y = x line y = x
iii. When x = 1 y = x the point lies on the y = x the point lies on the
line y = x line y = x
iv. When x > 1 y > x graph lies above the y < x graph lies below
line y = x the line y = x
Based on the above, the two graphs are shown in Figure 6.7. Note that if (a, b), (where a and
2
1/2
b are + ve) is a point on y = x , then (b, a) is a point on y = x . Hence, the graphs of the two
functions are symmetric about the line y = x.
Figure 6.7

2

1/2
1

0 1

Example
2
Show that the function y = x – 6x – 3 is symmetric about the line x = 3. Draw a broad graph of the
function. What is the domain and of the function?
Solution:
A function y = f(x) is symmetric about the line x = 3 if f(3 + k) = f(3 – k) for all real values of k.
Now f(3 + k) = (3 + k) – 6(3 + k) – 3
2
= 9 + 6k + k – 18 – 6k – 3
2
2
= k – 12
f(3 – k) = (3 – k) – 6(3 – k) – 3
2
= 9 – 6k + k – 18 + 6k – 3
2
2
= k – 12.

162 LOVELY PROFESSIONAL UNIVERSITY