Page 166 - DMTH201_Basic Mathematics-1

Unit 6: Functions

Notes
x
f f ( )
x
( ) = , g(x) 0
x
g g ( )
The domain of each of the resultant function is given by the intersection of the domains of f and
g. In the case of quotient, the value of x at which g(x) = 0 must be excluded from the domain.

Example
Examine whether the following functions are even or odd.

1 1
3
(a) y = x 2 (b) y 2 (c) y = x (d) y
x x
Draw the graph of each function.
Solution:
2
2
2
2
(a) Let f(x) = x , then f(–x) = (x) = x = f(x) y = x is an even function. This function is
symmetric about y-axis.
To draw graph, we note that when x = 0, then y = 0. Also y increases as x increases. The
graph of the function is shown in Figure 6.2.
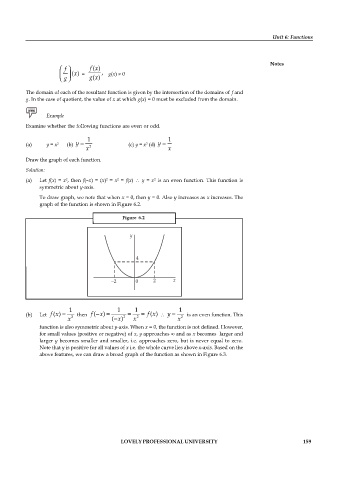
Figure 6.2

4

2 0 2

1 1 1 1
x
(b) Let ( )f x 2 then (f ) x 2 2 f ( ) y 2 is an even function. This
x ( x ) x x
function is also symmetric about y-axis. When x = 0, the function is not defined. However,
for small values (positive or negative) of x, y approaches and as x becomes larger and
larger y becomes smaller and smaller, i.e. approaches zero, but is never equal to zero.
Note that y is positive for all values of x i.e. the whole curve lies above x-axis. Based on the
above features, we can draw a broad graph of the function as shown in Figure 6.3.

LOVELY PROFESSIONAL UNIVERSITY 159