Page 317 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes

Notes
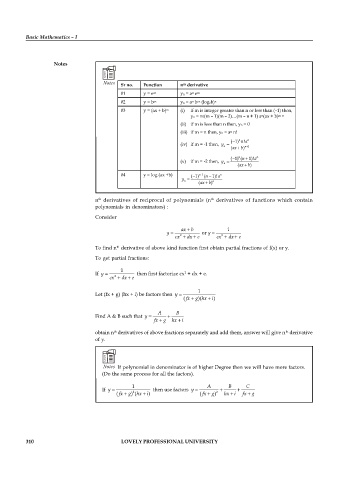
th
Sr no. Function n derivative
01 y = e yn = a e
n
ax
ax
n
ax
02 y = b yn = a b (logeb)
ax
n
03 y = (ax + b) (i) if m is integer greater than n or less than (–1) then,
m
yn = m(m – 1)(m – 2)…(m – n + 1) a (ax + b) m-n
n
(ii) if m is less than n then, yn = 0
(iii) if m = n then, yn = a n!
n
n
( 1) n !a n
(iv) if m = -1 then, y
n n 1
(ax b )
n
( 1) (n 1)!a n
(v) if m = -2 then, y n
(ax b )
04 y = log (ax +b) ( 1) n 1 (n 1)!a n
y n
(ax b ) n
th
th
n derivatives of reciprocal of polynomials (n derivatives of functions which contain
polynomials in denominators) :
Consider
ax b 1
y or y
cx 2 dx e cx 2 dx e
To find n derivative of above kind function first obtain partial fractions of f(x) or y.
th
To get partial fractions:
1
2
If y then first factorize cx + dx + e.
cx 2 dx e
1
Let (fx + g) (hx + i) be factors then y
( fx g )(hx i )
A B
Find A & B such that y
fx g hx i
th
th
obtain n derivatives of above fractions separately and add them, answer will give n derivative
of y.

Notes If polynomial in denominator is of higher Degree then we will have more factors.
(Do the same process for all the factors).
1 A B C
If y then use factors y
2
( fx g ) (hx i ) ( fx ) g 2 hx i fx g

310 LOVELY PROFESSIONAL UNIVERSITY