Page 57 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

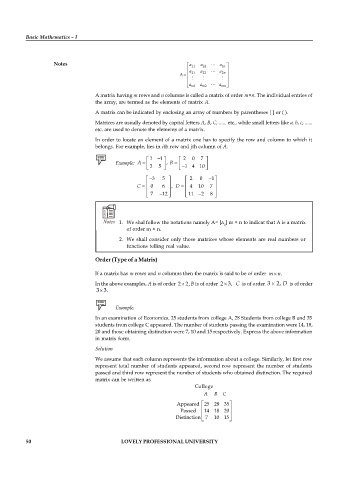
Notes a 11 a 12  a 1 n

a 21 a 22 a 2 n
A
  
a m1 a m2  a mn
A matrix having m rows and n columns is called a matrix of order m×n. The individual entries of
the array, are termed as the elements of matrix A.
A matrix can be indicated by enclosing an array of numbers by parentheses [ ] or ( ).
Matrices are usually denoted by capital letters A, B, C, ...... etc., while small letters like a, b, c, ......
etc. are used to denote the elements of a matrix.
In order to locate an element of a matrix one has to specify the row and column to which it
belongs. For example, lies in ith row and jth column of A.
1 1 2 0 7
Example: A , B
2 5 1 4 10

3 5 2 0 1
C 0 6 , D 4 10 7
7 12 11 2 8

Notes 1. We shal follow the notations namely A= [a ] m × n to indicat that A is a matrix
ij
of order m × n.
2. We shall consider only those matrices whose elements are real numbers or
functions telling real value.
Order (Type of a Matrix)

If a matrix has m rows and n columns then the matrix is said to be of order m . n
In the above examples, A is of order 2 , 2 B is of order 2 , 3 C is of order 3 , 2 D is of order
3 . 3

Example.
In an examination of Economics, 25 students from college A, 28 Students from college B and 35
students from college C appeared. The number of students passing the examination were 14, 18,
20 and those obtaining distinction were 7, 10 and 15 respectively. Express the above information
in matrix form.
Solution
We assume that each column represents the information about a college. Similarly, let first row
represent total number of students appeared, second row represent the number of students
passed and third row represent the number of students who obtained distinction. The required
matrix can be written as
College
A B C
Appeared 25 28 35
Passed 14 18 20
Distinction 7 10 15

50 LOVELY PROFESSIONAL UNIVERSITY