Page 62 - DMTH201_Basic Mathematics-1

Unit 3: Matrix

Notes
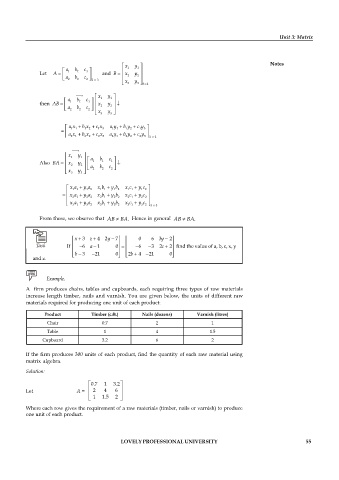
x y
a b c 1 1
Let A 1 1 1 and B x 2 y 2
a 2 b 2 c 2 2 3
x
3 y 3 3 2
x y
a 1 b 1 c 1 1 1
then AB x 2 y 2
a 2 b 2 c 2
x y
3 3
a x b x c x a y 1 b y 2 c y 3
1 2
1 3
1
1
1 1
1
a x b x 2 c x 3 a y 1 b y 2 c y 3 2 2
2
2
2 1
2
2
2
x 1 y 1
a b c
Also BA x 2 y 2 a 1 b 1 c 1
x 3 y 3 2 2 2
x a y a x b y b x c y c
1 1 1 2 1 1 1 2 1 1 1 2
x a y a x b y b x c y c
2 1 2 2 2 1 2 2 2 1 2 2
x a y a x b y b x c y c
3 1 3 2 3 1 3 2 3 1 3 2 3 3
From these, we observe that AB BA . Hence in general AB BA .
x 3 z 4 2y 7 0 6 3y 2
Task If 6 a 1 0 6 3 2c 2 find the value of a, b, c, x, y
b 3 21 0 2b 4 21 0
and z.

Example.
A firm produces chairs, tables and cupboards, each requiring three types of raw materials
increase length timber, nails and varnish. You are given below, the units of different raw
materials required for producing one unit of each product:
Product Timber (c.ft.) Nails (dozens) Varnish (litres)
Chair 0.7 2 1
Table 1 4 1.5
Cupboard 3.2 6 2

If the firm produces 300 units of each product, find the quantity of each raw material using
matrix algebra.
Solution:

0.7 1 3.2
Let A = 2 4 6
1 1.5 2

Where each row gives the requirement of a raw materials (timber, nails or varnish) to produce
one unit of each product.

LOVELY PROFESSIONAL UNIVERSITY 55