Page 61 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
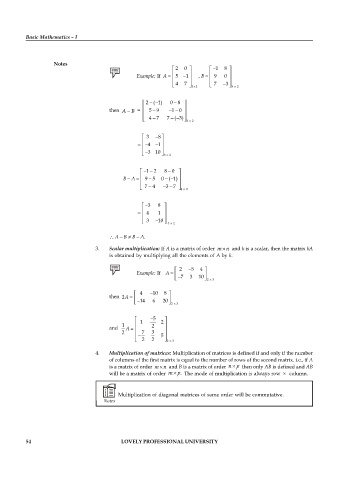
2 0 1 8
Example: If A 5 1 , B 9 0
4 7 7 3
3 2 3 2

2 ( 1) 0 8
then A B = 5 9 1 0
4 7 7 ( 3)
3 2

3 8
4 1
3 10
3 2

1 2 8 0
B A 9 5 0 ( 1)

7 4 3 7
3 2
3 8
4 1
3 10
3 2

A B B . A
3. Scalar multiplication: If A is a matrix of order m n and k is a scalar, then the matrix kA
is obtained by multiplying all the elements of A by k.
2 5 4
Example: If A
7 3 10
2 3

4 10 8
then 2A
14 6 20
2 3
5
1 2
1 2
and A
2 7 3
5
2 2 2 3
4. Multiplication of matrices: Multiplication of matrices is defined if and only if the number
of columns of the first matrix is equal to the number of rows of the second matrix. i.e., if A
is a matrix of order m n and B is a matrix of order n p then only AB is defined and AB
will be a matrix of order m . p The mode of multiplication is always row column.

Multiplication of diagonal matrices of same order will be commutative.
Notes

54 LOVELY PROFESSIONAL UNIVERSITY