Page 16 - DMTH202_BASIC_MATHEMATICS_II

Unit 1: Integration

Notes
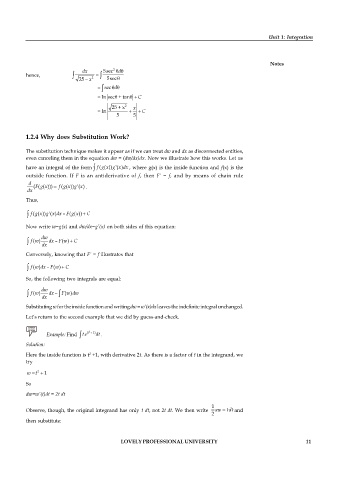
2
dx 5sec  d

hence,  2 
25 x 5sec


  sec d
 In sec + tan  C

25 + x 2 x
 In   C
5 5

1.2.4 Why does Substitution Work?

The substitution technique makes it appear as if we can treat dw and dx as disconnected entities,
even canceling them in the equation dw = (dw/dx)dx. Now we illustrate how this works. Let us
have an integral of the form f ( ( )) '( )dx , where g(x) is the inside function and f(x) is the

g
x
g
x
outside function. If F is an antiderivative of f, then F’ = f, and by means of chain rule
d
g
g
x
x
g
x
F
( ( ( )))  f ( ( )) '( ) .
dx
Thus,
x
x
F
g
x
 f ( ( )) '( )dx – ( ( )) C
g
g
Now write w=g(x) and dw/dx=g’(x) on both sides of this equation:
dw
w
w
F
 f ( ) dx – ( ) C
dx
Conversely, knowing that F’ = f illustrates that
w
w
F
 f ( )dx – ( ) C
So, the following two integrals are equal:
dw
 f ( ) dx – F ( )dw
w

w
dx
Substituting w for the inside function and writing dw = w’(x)dx leaves the indefinite integral unchanged.
Let’s return to the second example that we did by guess-and-check.
Example: Find t e (t 2  1) dt .

Solution:
2
Here the inside function is t +1, with derivative 2t. As there is a factor of t in the integrand, we
try
w t 2  1
So
dw=w’(t)dt = 2t dt
1
Observe, though, the original integrand has only t dt, not 2t dt. We then write aw  tdt and
2
then substitute:
LOVELY PROFESSIONAL UNIVERSITY 11