Page 170 - DMTH202_BASIC_MATHEMATICS_II

Unit 12: Permutation

Thus, Notes
P(6, 6) = 6!/2!3! = (6×5×4×3×2×1)/(2×1)(3×2×1) = (6×5×4)/2 = 60 (not 6!).

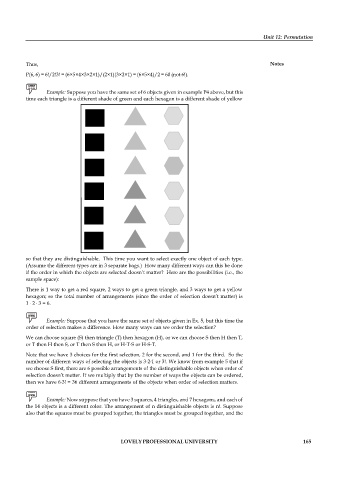
Example: Suppose you have the same set of 6 objects given in example P4 above, but this
time each triangle is a different shade of green and each hexagon is a different shade of yellow

so that they are distinguishable. This time you want to select exactly one object of each type.
(Assume the different types are in 3 separate bags.) How many different ways can this be done
if the order in which the objects are selected doesn’t matter? Here are the possibilities (i.e., the
sample space):
There is 1 way to get a red square, 2 ways to get a green triangle, and 3 ways to get a yellow
hexagon; so the total number of arrangements (since the order of selection doesn’t matter) is
1 2 3 = 6.

Example: Suppose that you have the same set of objects given in Ex. 5, but this time the
order of selection makes a difference. How many ways can we order the selection?

We can choose square (S) then triangle (T) then hexagon (H), or we can choose S then H then T,
or T then H then S, or T then S then H, or H-T-S or H-S-T.
Note that we have 3 choices for the first selection, 2 for the second, and 1 for the third. So the
number of different ways of selecting the objects is 321 or 3!. We know from example 5 that if
we choose S first, there are 6 possible arrangements of the distinguishable objects when order of
selection doesn’t matter. If we multiply that by the number of ways the objects can be ordered,
then we have 63! = 36 different arrangements of the objects when order of selection matters.

Example: Now suppose that you have 3 squares, 4 triangles, and 7 hexagons, and each of
the 14 objects is a different color. The arrangement of n distinguishable objects is n!. Suppose
also that the squares must be grouped together, the triangles must be grouped together, and the

LOVELY PROFESSIONAL UNIVERSITY 165